Thrust Required for Level Flight

If Eq. 9.4 is divided by Eq. 9.5, we find that the ratio of thrust required to total air­plane weight is equal to the ratio of the drag coefficient to the lift coefficient, or:

T = TR = thrust required = – jjD ’

which illustrates again the importance of the L/D ratio in performance character­istics. That is, to minimize the amount of thrust that must be used in level flight, the L/D ratio must be as large as possible. It is useful to determine how the thrust required varies with flight speed; Eq. 9.3 provides the needed information. First, we calculate the required lift coefficient to provide the lift to balance the weight as a function of flight speed:

C = _W = 2W

Cl qS pv2s •

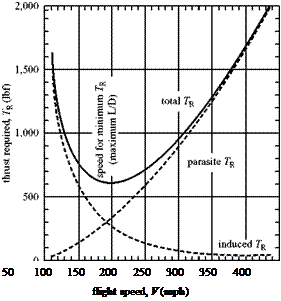
Figure 9.4. Thrust required for Bf-109G.

Substituting this result into Eq. 9.3 gives the drag coefficient as a function of flight speed; namely:

Notice that the induced-drag coefficient decreases rapidly (as the inverse fourth power of the speed) as V increases. This indicates that induced drag is most impor­tant at lower speeds, whereas the parasite drag dominates at high speed. The thrust required as a function of velocity is found readily by means of Eq. 9.4. We find:

This is plotted in Fig. 9.4 for the Messerschmitt Bf-109G. All values shown are for standard sea-level atmospheric conditions. (The changes that result from higher alti­tudes are checked in an exercise at the end of the chapter.) Both the induced-and parasite-drag contributions are plotted separately and compared to the total thrust required.