Properties of angular velocities

The angular velocity tensor and vector have additive properties. Their superscripts reveal the rotational direction, and they relate to coordinate transformations in a special way.

Property 1

Angular velocities are additive. For example, if frame В revolves relative to frame A with ttBA and frame C wrt frame В with flCfl, then C revolves wrt A with the angular velocity tensor

(4.58)

The vector equivalent is

(4.59)

The superscripts reflect the sequence of addition. By contracting adjacent letters, you get the result on the left-hand side.

Proof: Apply the Euler transformation twice to an arbitrary vector x Da x = DB x + ПВАх – DB x = Dcx + Псвх and substitute the second into the first equation:

Da x = Dc x + Псвх + ПВАх = Dc x + (Псв + ПВА)х Compare this result with a third application of Euler’s transformation

Da x = Dcx + ПСАх and conclude: flCA = flCft + ПВА.

Property 2

Reversing the sequence between two frames changes the sign of the angular velocity tensor:

nBA = – nAB

The vector equivalent is

Proof: Replace C by A in Eq. (4.58) and, because flAA = 0, the relationship is proven. The angular velocity vector equivalent follows by contraction.

Property 3

The rotational time derivative of the angular velocity vector between two frames can be referred to either frame

Daujba = Dbuba (4.62)

Proof: Transform the rotational time derivative of шВА from frame A to В,
Daujha = Dbujba + ПВАшВА

and recognize that the last term, being the vector product of the same vector, is zero.

Note that Eq. (4.62) also holds for regular time derivatives expressed in the associated coordinate systems ]A and ]B:

Proof: From Eq. (4.62)

[Dacoba]a = [T]ab[Dbcoba]b

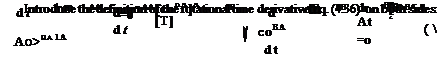which proves the relationship.

Property 4

The coordinated angular velocity can be calculated from the coordinate trans­formations of the associated coordinate systems:

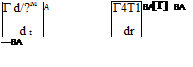Proof: From the definition of the angular velocity tensor, Eq. (4.47) expressed in the associated coordinate system ]A and because of Eq. (4.6) we form

The second part is proven by transforming to the ]B coordinate system

Example 4.10 Turbojet Spooling

Problem. A pilot increases thrust as he pulls up the aircraft. The turbine’s Г angular velocity [coTA]A wrt the airframe A is recorded in airframe coordinates l’1, and the aircraft pitch-up rate coAEL wrt Earth E is measured by the onboard INS in local-level coordinates ]L . Both angular velocities are changing in time. Determine the angular acceleration of the turbine [АшТЕ/At]1 wrt Earth in local – level coordinates. The INS provides also the direction cosine matrix [ T |’u.

Solution. The solution follows the two-step approach: Solve the problem in tensor form, followed by coordination. We use the additive property of angular ve­locities then apply the rotational derivative wrt Earth and the Euler transformation to recover the turbine revolutions-per-minute measurement. Then we introduce the appropriate coordinate systems to present the turbine acceleration in local-level coordinates.

The angular velocity of the turbine wrt Earth is

Take the rotational derivative wrt Earth

Deute = Deuta + DeuAe

Transform the turbine derivative DEwTA to the airframe A and obtain DEuTE = DAuTA + Оаешта + Deljae

Introduce local-level coordinates

[DEa)TE]L = [DAa)TA]L + [QAEf[coTA]L + [DEa, AE]L

Because the local-level coordinate system is associated with the Earth frame, the first and last rotational derivative simplify to the ordinary time derivative. The second rotational derivative, as well as the turbine speed, must be transformed to the airframe axis to get the desired result:

Г rmTE 1	L	d coTA	A	Г dcuA£"|
	= [T]AL		+ [Q. AEf[T]AL[ioTA]A +
dt		dt		dt

As you see, you cannot just add together the angular accelerations. The turbine speed also couples with the aircraft angular rate as an additional acceleration term.

Sections 4.1 and 4.2 that you have just mastered are the trailblazers for invariant modeling. Particularly, the rotational time derivative and the Euler transformation preserve the tensor characteristics of kinematics, even under time-dependent co­ordinate transformations. To adopt them as your own, and apply them whenever the opportunity arises, should become your ambition. I have put them to good use for 30 years, and they spared me some major headaches.