Angular Momentum

The angular momentum is the cousin of linear momentum. If you multiply the linear momentum by a displacement vector, you form the angular momentum. That at least is true for particles. By summing over all of the particles of a body, we define its total angular momentum. Again, introducing the c. m. will not only enable a compact formulation and simplify the change of reference points, but will also justify the separate treatment of attitude and translational motions. We close out this section with the formula for clusters of bodies, both for the common c. m. and an arbitrary reference point.

6.2.1 Definition of Angular Momentum

The definition of the angular momentum follows a pattern we have established for the linear momentum (Sec. 5.1). We start with a single particle and then em­brace all of the particles of a particular body. Rigid-body assumptions and c. m. identification will lead to several useful formulations.

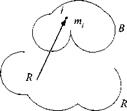
To define the angular momentum of a particle, we have to identify two points and one frame: the particle i, the reference point R, and its reference frame R (see Fig. 6.7).

Definition: The angular momentum lfR of a particle і with mass m, relative

to the reference frame R and referred to reference point R is defined by the vector product of the displacement vector siR and its derivative DRsm multiplied by its

mass M;:

І ж = miSiRDRsiR = miSiRvf (6.15)

Because the rotational derivative of s, s is the linear velocity of the particle, DRslR = vf and mtvf — pf is the linear momentum; we can express the angular momentum simply as the vector product of the displacement tensor and the linear momentum

lfR = SmP* (6-16)

The direction of the angular momentum is normal to the plane subtended by the displacement and the linear momentum vectors. (Any particle that is not at rest has a linear and angular momentum; it is just a matter of perspective. If the reference consists only of a frame, it exhibits linear momentum properties only. If a reference point is introduced, it displays also angular momentum characteristics.)

A body B, not necessarily rigid, can be considered a collection of particles і. The angular momentum of this body В relative to the reference frame R and referred to the reference point R is defined as the sum over the angular momenta of all particles

if = Yl* = Ym’s‘«DRs’« = YmiSiRVf = Ys‘rp’ (6Л7)

і І І і

Notice the shift of the subscript і in JA lfR to a superscript В in lBR, reflecting the gathering of all particles into body B.