Developing a test philosophy using the free-air method

As already mentioned tip effect evaluations consist of establishing a series of hovers so that the variation of power (referred) with RRPM (referred) at constant weight (referred) can be documented. It is important, therefore, that the appropriate test philosophy is used to ensure that an adequate range of test data is obtained. Consider the situation that arises when a test team elects to establish a series of hovers and during each hover they vary the RRPM by +1 rad/s from nominal. (They conduct a rotor sweep.) Assume for simplicity that all the hovers are conducted at the same height (but at different weights) and that the fuel burn during each rotor sweep can be ignored. Figure 3.18 shows a typical set of raw data. The team then processes the data to yield the required referred parameters, see Fig. 3.19. Any assessment of the importance of tip effects has been complicated since the actual weight at which each rotor sweep was conducted has yielded three referred weights as shown by the three highlighted points in each figure. In practice, due to the uncertainties associated with actual flight test, the team will usually be unsure if the differences in referred power are due solely to tip effects. The correct test philosophy, therefore, is to plan a series of hovers at differing weights and altitudes that will generate a consistent set of referred weights.

As an example of the correct approach to tip effect evaluation consider the planning required for a single test point based on a nominal condition of 4000 kg at 5000 ft (ISA) and a rotor sweep of 95% to 105%. At 5000 ft the relative density is 0.8617 so the referred weight resulting from the nominal conditions (100% RRPM) is 4000/ 0.8617 = 4642 kg. Suppose sometime later the weight has reduced by 50 kg to 3950 kg and the team wish to establish the 95% RRPM test point. Knowing that the

Fig. 3.19 Hover performance data – referred.

referred weight required is 4642 kg and that m will be 0.95 (m2 = 0.9025) it is possible to determine the relative density required to give the desired referred weight when the actual weight is 3950 kg:

This relative density will be found at around 2000 ft. Likewise, if later in the sortie when the helicopter weight reaches 3900 kg, the team wish to establish the 105% RRPM a test point at an altitude of approximately 9000 ft would be required to generate the desired referred weight. It should be clear, therefore, that an accurate estimate of air density (OAT at regular pressure altitudes) is an important factor in successful planning.

So far in this discussion actual RRPM has been considered as the controlling parameter. In fact the test objective is to assess the effect on referred power of referred RRPM (m/^6) at constant referred weight. To allow for data interpolation, and possible extrapolation, it will be necessary for the test team to gather data from a series of test points over a range of referred weights with at least two values of referred RRPM established at each referred weight. Since OAT and therefore V6 will vary with altitude the variation of the actual RRPM required to meet the test objectives will need to be determined and compared with any published limitations. The example introduced above will be used to illustrate this point. Assume that the required referred

RRPM range is 0.95 to 1.05 (equating to 95% RRPM to 105% RRPM at sea level ISA) and that the nominal rotor RPM is 100%. Since in this example the temperature profile is known it is possible to use:

W, W x JL, W, 4642

amz § § m /

Thus for the three referred RRPMs required (0.95, 1.00 and 1.05) a value of W/§ can be found which will dictate a particular pressure altitude for a given weight. For example, if a/V9 equals 1.00 and the AUM is 4000 kg, then:

and

4000

4642

This relative pressure can be found at approximately 4100 ft Hp. At this altitude the OAT will be 280 K and therefore the required RRPM for a/V9 = 1.00 is given by:

Thus an actual RRPM of 98.6% will be required at 4100 ft to generate the desired referred RRPM and weight. Similarly if a/V9 = 0.95 is set when the AUM is 3950 kg a pressure altitude of 1600 ft and RRPM of 94.5% will be required. Finally if a/V9 = 1.05 is desired at 3900 kg a height of 7300 ft and RRPM of 102.3% will be needed.

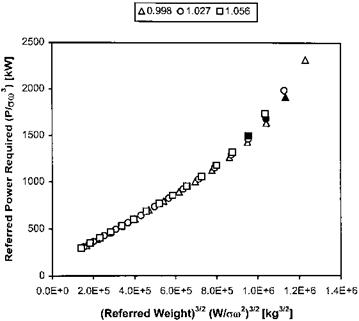
In order to meet all the test objectives it is therefore evident that the test team must plan each sortie in detail. It is usual to use altitude to generate changes in referred weight as well as changing altitude to maintain the desired referred weight for different referred RRPMs. If a sensible choice of referred weight(s) is made it may be possible to maintain hover height and change rotor speed thereby generating a test point at a different referred weight which will be completed at some other altitude. A sample set of test points is shown in Table 3.9 and an example plot of the relevant referred data

Table 3.9 Typical vertical performance test point matrix. Start mass (kg) Fuel gone (kg) Hp (ft) OAT (°С) RRPM (%) W/jaL (kgf) ю/%9 4750 50 8000 -0.8 102.1 5750 1.05 4750 75 5100 4.9 97.2 5750 0.99 4750 100 6900 1.3 99.6 5760 1.02 4750 125 4500 6.1 100.4 5240 1.02 4750 150 7400 0.3 102.3 5500 1.05 4750 175 3300 8.5 97.9 5260 0.99 4750 200 6300 2.5 99.8 5510 1.02 4750 225 6700 1.7 102.6 5260 1.05 4750 250 4900 5.3 97.3 5490 0.99

Fig. 3.20 Referred hover data – correct methodology.

is at Fig. 3.20. Note that it is now possible to make a judgement on the magnitude of any tip effects.