Disturbed flapping motion at a constant feather angle

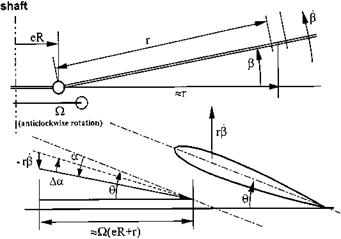
The rotor blades are assumed to be at a constant feather angle and the rotor is taken as rotating steadily at an angular velocity ). Since it is only the disturbed motion that is of interest the aerodynamic moment corresponding to the feather angle will be ignored. When the blade flaps up with angular velocity p, there is a relative down velocity of rp at a point on the blade located r from the hinge, see Fig. 4.7. From Fig. 4.7 it can be seen that for small angles of inflow, flap and flap disturbance:

— rp

Да в tan Да в 4

)(eR + r)

Thus the change in elemental lift, SL, is given by:

1 1 rR 1

SL = 2 pV2c SrCL = —2 p )2(r + eR)2ca r + ^ Sr = —^ p )r(r + eR)pca 8r

Now the aerodynamic moment is obtained by integrating the lift over the blade:

mi – e) mi- e) і

MA = I r dL = — I 2 P 2(r + eR)$ca dr

Jo Jo

= —1 p Qac p R4(1 — e)3(1 + e/3)

8

Therefore:

Mk у . _

T1 =—У )P(1 — e)3(1 + e/3) where:

pacR4

у = —-— = Lock’s inertia number

*yy

Therefore using Equations (4.19) and (4.20) the flapping equation becomes:

p + У )(1 + e)3(1 + e/3)p + )2(1 + g)p = 0 8

Taking Laplace transforms:

^2 + У )(1 — e)3(1 + e/3)s + )2(1 + g) = 0 8

The above second-order equation relates directly to the standard form:

s2 + 2^mn s + m2 = 0

Hence:

у(1 — e)3(1 + e/3)

16V1 + s

The motion described above is damped and harmonic in nature with a natural frequency, mn, of )V1 + s. If the rotor has no flapping hinge offset then both e and s will be zero. Therefore the natural frequency is exactly equal to the shaft frequency and consequently the flap mode is being forced at its resonant frequency; therefore it will display a phase shift of 90° between feather (the input) and flap (the output).