Effect of airspeed on dynamic modes

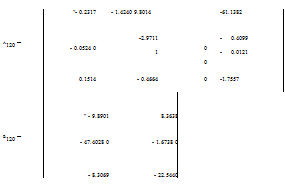
An estimate of the likely behaviour can be made by examining the aero-derivative and control matrices. Below are a set of matrices for three flight cases: hover, 60KTAS and 120KTAS. Note that the yaw angle response has been removed and that the state vector has been re-ordered to [v, p, ф, r]T.

" – 9.7060 5.6145"

– 47.0134 – 1.1236

0 0 – 8.4807 – 15.1333

– 0.1320	0.9829	9.7929	-30.4417
– 0.0455	– 3.0478	0	– 0.2221
0	1	0	0.0535
0.1016	– 0.4899	0	– 1.1085

A60 —

Again these eigenvalues imply three stable modes. In this case the second-order mode has a natural frequency of 1.833 rad/s giving an observed period of 3.6 s and a relative damping value of 0.291 (T1/2 of 1.3 s). The first-order responses have time constants of 19.69s ( — 0.0508) and 0.32s ( — 3.1717).

(3) 120 KIAS. The eigenvalues of the A120 matrix are:

— 0.8882 + 2.9488г, — 0.8882 — 2.9488г, — 0.0528, — 3.1292

Here the second-order mode has a natural frequency of 3.08 rad/s giving an observed period of 2.1s and a relative damping value of 0.288 (T1/2 of 0.8 s). The first-order responses have time constants of 18.94s ( — 0.0528) and 0.32s ( — 3.1292).

Thus the Lateral/Directional Oscillation (LDO) is stable throughout the speed range although the period and damping of the oscillatory mode varies with airspeed. The helicopter would appear, therefore, dynamically stable to the pilot with the frequency increasing with airspeed. This result agrees with detailed analysis given above. Also, since the spiral mode is characterized by the first order mode with the longer time constant, note that for the example helicopter the mode is stable with the rate of convergence being greater at 120 KIAS than at 60 KIAS. The roll control response is, therefore, characterized by the other, shorter, first-order mode and for the example helicopter there appears to be very little change in this mode with airspeed.

4.11.7.1 Effect of increased roll damping

The effect of an increase in roll damping, achieved by a direct increase in the numerical value of the rolling moment due to roll rate (Lp), can be shown by increasing the appropriate value in the A matrix. Consider the 60 knot case discussed earlier: and suppose the value of Lp is increased from — 3.0478 to — 6.0956:

" — 0.1320	0.9829 9.7929	— 30.4417
— 0.0455	— 6.0956	0	— 0.2221
0	1	0	0.0535
0.1016	— 0.4899	0	— 1.1085_
" — 9.7054	5.7541
— 47.0742	— 1.1515
0	0
— 8.3750	— 15.5094

A60 —

B60 —

The effect of this change on the dynamic response can be seen from the change in the eigenvalues:

(1) Before. — 0.5329 + 1.7540i, — 0.5329 — 1.7540i, — 0.0508, — 3.1717, resulting in the following engineering parameters:

C — 0.291, mn — 1.833 rad/s, T1 — 19.69 and T2 — 0.32

(2) After. – 0.5892 + 1.7384г, – 0.5892 – 1.7384г, – 0.0183, – 6.1393, resulting in the following:

C = 0.321, mn = 1.836 rad/s, T1 = 54.54 and T2 = 0.163

This suggests that increasing Lp hardly affects the LDO but will shorten the time constant of the roll mode. In addition, the spiral mode will have reduced stability since it will take much longer for the helicopter to return to wings level flight following a pulse input of lateral cyclic. It is interesting to note that a doubling of the rate damping has halved the time constant thereby improving the control predictability by reducing the time to achieve a steady roll rate.

4.11.7.2 Effect of increased yaw damping

As with the roll damping example, the effect of increasing Nr can be seen by changing the numerical value of the appropriate matrix element. Suppose the value of Nr is increased from – 1.1085 to – 4.4340:

	"- 0.1320	0.9829 9.7929	– 30.4417
о © II	– 0.0455	– 3.0478	0	– 0.2221
0	1	0	0.0535
B60 =	0.1016 – 9.7054 – 47.0742 0 – 8.3750	– 0.4899 5.7541 – 1.1515 0 – 15.5094	0	– 4.4340

The effect of this change on the dynamic response can be seen from the eigenvalues of the modified matrix: – 3.9231, – 2.5885, – 0.8745, – 0.2277, resulting in the following modal characteristics: T1 = 0.255, T2 = 0.386, T3 = 1.144 and T4 = 4.392. These results suggest that increasing Nr has a major effect on both the LDO and the spiral mode. The LDO has degenerated into two convergent first-order responses implying that it has been completely suppressed. In addition, the time constant of the spiral mode has been reduced thereby implying significant strengthening of the spiral stability.

4.11.7.3 Effect of increased lateral static stability

As with longitudinal stability the static derivatives, in this case Lv and Nv, have a significant effect on the dynamic modes. Before studying the effect of increased dihedral effect (Lv) it would be instructive to surmise the likely results. If a helicopter has increased lateral static stability and all other derivatives are left unchanged then for a given amount of lateral velocity (sideslip) it will generate a larger moment away from the direction of the sideslip. This will reduce the time taken for the aircraft to roll wings-level following a pulse on lateral cyclic and will probably cause a more oscillatory LDO since the roll attitude will change more readily for the same variations in lateral velocity.

Changing the numerical value of the appropriate matrix element can check these deductions. Suppose Lv is changed from — 0.0455 to — 0.1820:

" — 0.1320	0.9829	9.7929	— 30.4417"
— 0.1820	— 3.0478	0	— 0.2221
0	1	0	0.0535
0.1016	— 0.4899	0	— 1.1085_
— 9.7054	5.7541
— 47.0742	— 1.1515
0	0
— 8.3750	— 15.5094

A60 —

B60 —

The effect of this change on the dynamic response can be seen from the eigenvalues of the modified matrix: — 0.3670 + 1.9618г, — 0.3670 — 1.9618г, — 0.1454 — 3.4079, resulting in the following: £ — 0.184, mn — 1.996 rad/s, T1 — 6.83 and T2 — 0.29. These results confirm the deductions above. An increase in lateral static stability reduces the time constant of the spiral mode from almost 20 s to below 7 s and causes, approxi­mately, a 40% reduction in the damping of the LDO.

4.11.7.4 Effect of increased directional static stability

If a helicopter has increased directional static stability and all other derivatives are left unchanged then for a given amount of lateral velocity (sideslip) it will generate a larger yawing moment towards the direction of the sideslip. This will serve to increase the time taken for the aircraft to roll wings-level following a pulse on lateral cyclic because there will be a stronger tendency to ‘turn-into-wind’. In addition, one would expect the LDO to be less oscillatory since there should be smaller variations in lateral velocity for the same size of input.

Once again changing the numerical value of the appropriate matrix element can check these deductions. Suppose Nv is changed from 0.1016 to 0.4064:

" — 0.1320	0.9829 9.7929	— 30.4417"
— 0.0455	— 3.0478	0	— 0.2221
0	1	0	0.0535
0.4064	— 0.4899	0	— 1.1085_
— 9.7054	5.7541
— 47.0742	— 1.1515
0	0
— 8.3750	— 15.5094

A60 —

B60 —

The effect of this change on the dynamic response can be seen from the modified eigenvalues: — 0.5837 + 3.4925г, — 0.5837 — 3.4925г, — 0.0184, 3.1025, resulting in the following engineering parameters: £ — 0.165, mn — 3.541 rad/s, T1 — 54.35 and T2 — 0.32. These results certainly confirm our deductions regarding the spiral mode. The time constant for this mode has increased by almost threefold from over 19 s to nearly 55 s.

Chapter 5