Bank-to-turn incidence angles and rates

9.1.2.3 Now we use two dif­ferent incidence angles. The included angle between the geographic velocity vector v f and the first unit vector of the body b i is the total angle of attack a (we maintain the same symbol as in the skid-to-turn case). It is contained in the lB, 3B vertical body plane, which is also called the normal load factor plane. The banking of this plane from the vertical plane lv,3V is designated by the bank angle фву (see Fig. 9.3). Distinguish carefully between the Euler roll angle фвс (body axes, see Chapter 3), the aerodynamic roll angle ф’ (aeroballistic axes, see Chapter 3), and our bank angle фВу (velocity axes).

The body axes ]B and the geographic velocity axes ]v are defined as before. However, the total angle of attack a lies now in the normal load factor plane, and the bank angle фв/ is obtained by rotating about the velocity vector v f, which is parallel to the base vector v i. The sequence of rotation is

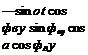and the transformation matrix of the body coordinates wrt the geographic velocity coordinates is

Here you can see the difference between the aeroballistic and bank angle treat­ments. Compare this transformation with the transformation matrix of the body wrt the aeroballistic wind coordinates [T]BV, Eq. (3.19) of Chapter 3, and you will recognize the difference.

The angular velocity of the body frame wrt the geographic velocity frame is derived from Fig. 9.3. Combining the incidence rates with their respective unit vectors and adding them vectorially yields

шву = 4>BVv+ab2 (9.12)

Expressed in body coordinates

[coBV]B = 4>BV[T}BV[Vl]v + a[b2]B and evaluated with the help of Eq. (9.11),

фВуcos a a

<pBV sin a

The angular velocity of the body frame wrt the geographic velocity frame is a function of the incidence angular rates and the angle of attack (redefined) but not of the bank angle. Both the angular rates and the angle of attack are given by the transfer functions of the autopilot.