Kutta-Joukowski Transformation

Kutta—Joukowski transformation is the simplest of all transformations developed for generating aerofoil shaped contours. Kutta used this transformation to study circular-arc wing sections, while Joukowski showed how this transformation could be extended to produce wing sections with thickness t as well as camber. In our discussions here, we make another simplification that the transformation is confined to the study of the actual contour of the circle, and to show how its shape changes on transformation.

In our discussion on Kutta—Joukowski transformation, it is important to note the following.

• The circle considered, in the physical plane, is a specific streamline. Essentially the circle is the stagnation streamline of the flow in the original plane 1 (z-plane).

• The transformation can be applied to the circle and all other streamlines, around the circle, to generate the aerofoil and the corresponding streamlines in plane 2 (f-plane) or the transformed plane. That is, the transformation can result in the desired aerofoil shape and the streamlines of the flow around the aerofoil.

It is convenient to use polar coordinates in the z-plane and Cartesian coordinates in f-plane. The Kutta—Joukowski transformation function is.

where b is a constant.

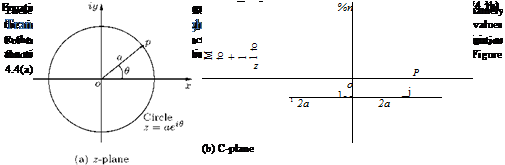
These § and n represent a straight line coinciding with the §-axis in the f-plane. The transformed line is thus confined to §-axis, as shown in Figure 4.4(b), and as в varies from 0 to n, point P moves from +2a to -2a. Thus, the chord of the locus of point P is 4a.

Note that the singularities at z = ± b produce sharp edges at Z = ± 2a. That is, the extremities of the straight line are sharp.