Trim Control Angles

Generally in a helicopter analysis, the value of Ct/g is known from its definition:

and thus the primary use of the equation for CT/o is to determine the collective pitch, 0O, which will be used in subsequent analysis:

3 3 2

The longitudinal cyclic pitch Bx has already been derived:

The magnitude of the flapping, alp is determined by the pitching moment that the rotor must produce to balance such things as an offset center-of-gravity position, a

lift force on the horizontal stabilizer, and/or an aerodynamic pitching moment on the fuselage. If the net of these effects is zero, then ax will also be zero. If the net effect is not zero, ax can be evaluated by the methods derived in Chapter 8, "The Helicopter in Trim.”

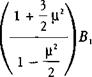For some types of problems, such as determining the stability and control characteristics of the rotor or for calculating the flapping of a tail rotor or of a rotor in a wind tunnel with fixed cyclic pitch, it is convenient to rewrite the equation in terms of the angle of attack of the plane perpendicular to the shaft, a„ rather than the angle of attack of the tip path plane. In this case:

The equation for the lateral cyclic pitch, Ax> may be derived by setting the pitching moment on the rotor to zero. The result is:

where the lateral flapping, bx , is that required to trim the helicopter for external rolling moments such as those produced by tail rotor thrust or a lateral center of gravity offset. For most steady flight conditions, bls can be considered to be negligible.