Transformation of a Circle to a Cambered Aerofoil

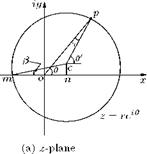
For transforming a circle to a cambered aerofoil, using Joukowski transformation, the center of the circle in the physical plane has to be shifted to a point in one of the quadrants. Let us consider the center in the first coordinate of z-plane, as shown in Figure 4.8(a).

Figure 4.8 Transformation of a circle to a cambered aerofoil.

As seen in Figure 4.8(a):

• the center c of the circle is displaced horizontally as well as vertically from the origin, in the physical plane.

• let the horizontal shift of the center be “on = be" and

• the vertical shift be “cn = h"

The point p on the circle and its distance from the origin can be represented as shown in Figure 4.9.

Both the vertical shift h and eccentricity e are small. Therefore, the angle в, subtended at point m, by om and cm is small, hence, cos в & 1, also cos у & 1. Therefore, the radius of the circle becomes:

a = (b + be) cos в = (b + be).

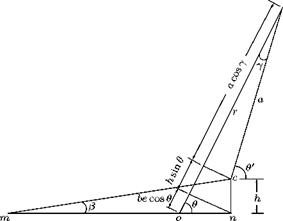
The vertical shift of the center can be expressed as:

h = a sin в = b (1 + е)в.

But e and в are small, therefore, h becomes:

h ^ Ьв

By dropping perpendiculars on to op, from n and c, it can be shown that: op = r = a cos у + h sin в + be cos в.

The angle y is small, therefore, cos y ^ 1, thus:

r = a + h sin в + be cos в. Substituting for a = (b + be) and h = Ьв, r becomes:

r = b + be + bв sin в + be cos в.

This can be expressed as:

r

– = 1 + e + e cos в + в sin в b

or

– = [1 + (e + e cos в + в sin в)]

Expanding and retaining only the first order terms, we have:

– = 1 — e — e cos в — в sin в r

since e and в are small, their powers are assumed to be negligibly small. The Joukowski transformation function is:

b2

Z = Z + —.

z

Replacing z with r е’в, we have:

reie + – e-ie

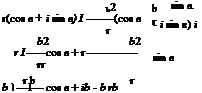r

rb

Substituting for — and – from Equations (4.7a) and (4.7b), we get: br

Z = 2b cos в + i2b(e + e cos в + в sin в) sin в

or

Z + in = 2b cos в + i2b(e + e cos в + в sin в) sin в.

Equating the real and imaginary parts, we get:

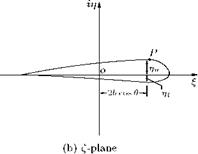
These coordinates represent a cambered aerofoil, in the Z-plane, as shown in Figure 4.8(b). Thus, the circle with center c in the first quadrant of z-plane is transformed to a cambered aerofoil section in the Z-plane, as shown in Figure 4.8(b), with coordinates Z and n, given by Equations (4.8a) and (4.8b). It is seen that:

• The chord of the cambered aerofoil is also 4b, as in the case of symmetrical aerofoil.

• When в = 0, that is, when there is no vertical shift for the center of the circle, in the physical plane, the transformation results in a symmetrical aerofoil in the transformed or Z-plane.

• The second term in the n expression, in Equation (4.8b), alters the shape of the aerofoil section, because it is always a positive addition to the n-coordinate (ordinate).

• The trailing edge is sharp (n = 0, at в = n), and the fmax is at the quarter chord point (Z = b).