Local-differential momentum theory and dynamic inflow

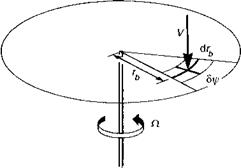
We begin by considering the simple momentum theory applied to the rotor disc element shown in Fig. 3.17. We make the gross assumption that the relationship between the change in momentum and the work done by the load across the element applies locally as well as globally, giving the equations for the mass flow through the element and the thrust differential as shown in eqns 3.161 and 3.162.

dm = pVrb drb df (3.161)

dT = dm 2vi (3.162)

Using the two-dimensional blade element theory, these can be combined into the form

2b ^2pa°c (9UT + UtUp) drb df 2 = 2ргь (V + (ki – )2) 1 ki drb df

(3.163)

Fig. 3.17 Local momentum theory applied to a rotor disc

Integrating around the disc and along the blades leads to the solution for the mean uniform component of inflow derived earlier. If, instead of averaging the load around the disc, we apply the momentum balance to the one-per-rev components of the load and inflow, then expressions for the non-uniform inflow can be derived. Writing the first harmonic inflow in the form

Xi = X0 + rb(Mc cos ф + Xs sin ф) (3.164)

eqn 3.163 can be expanded to give a first harmonic balance which, in hover, results in the expressions

3a0s f (1) 16 X0 F1c
(3.165)
X1c
and
3a0s F(1) 16 X0 1s
X1s	(3.166)

where the F loadings are given by eqns 3.92 and 3.93. These one-per-rev lift forces are closely related to the aerodynamic moments at the hub in the non-rotating fuselage frame – the pitching moment Сма and the rolling moment Cpa, i. e.,

2CLa aos	A F (1) 8 F1s
(3.167)

2CMa _3 f (1)

a0 S = 8 F1c

These hub moments are already functions of the non-uniform inflow distributions; hence, just as with the rotor thrust and the uniform inflow, we find that the moments are reduced by a similar moment deficiency factor

CLa = C1 CLaQS (3.169)

(3.170)

(3.171)

in hover, with typical value 0.6, and

1

1 1 00£

1 + 8n

in forward flight, with typical value of 0.8 when д = 0.3. In hover, the first harmonic inflow components given by eqns 3.165 and 3.166 can be expanded as

Ms = C1-^-(91S + в1с + p)

16A0

As the rotor blade develops an aerodynamic moment, the flowfield responds with the linear, harmonic distributions derived above. The associated deficiency factors have often been cited as the cause of mismatches between theory and test (Refs 3.9,3.22­3.29), and there is no doubt that the resulting overall effects on flight dynamics can be significant. The assumptions are fragile however, and the theory can, at best, be regarded as providing a very approximate solution to a complex problem. More recent developments, with more detailed spatial and temporal inflow distributions, are likely to offer even higher fidelity in rotor modelling (see Pitt and Peters, Ref 3.26, and the series of Peters’ papers from 1983, Refs 3.27-3.29).

The inflow analysis outlined above has ignored any time dependency other than the quasi-steady effects and harmonic variations. In reality, there will always be a transient lag in the build-up or decay of the inflow field; in effect, the flow is a dynamic element in its own right. An extension of momentum theory has also been made to include the dynamics of an ‘apparent’ mass of fluid, first by Carpenter and Fridovitch in 1953 (Ref. 3.30). To introduce this theory, we return to axial flight; Carpenter and Fridovitch suggested that the transient inflow could be taken into account by including an accelerated mass of air occupying 63.7% of the air mass of the circumscribed sphere of the rotor. Thus, we write the thrust balancing the mass flow through the rotor to include an apparent mass term

inflow is therefore very rapid, according to simple momentum considerations, but this estimate is clearly a linear function of the ‘apparent mass’. Since this early work, the concept of dynamic inflow has been developed by a number of researchers, but it is the work of Peters, stemming from the early Ref. 3.23 and continuing through to Ref. 3.29, that has provided the most coherent perspective on the subject from a fluid mechanics standpoint. The general formulation of a 3-DoF dynamic inflow model can be written in the form

The matrices M and L are the apparent mass and gain functions respectively; Ct , Cl and Cm are the thrust, rolling and pitching aerodynamic moment perturbations inducing the uniform and first harmonic inflow changes. The mass and gain matrices can be derived from a number of different theories (e. g., actuator disc, vortex theory). In the most recent work, Peters has extended the modelling to an unsteady three­dimensional finite-state wake (Ref. 3.29) which embraces the traditional theories of Theordorsen and Lowey (Ref. 3.31). Dynamic inflow will be discussed again in the context of stability and control derivatives in Chapter 4, and the reader is referred to Refs 3.28 and 3.29 for full details of the aerodynamic theory.

Before discussing additional rotor dynamic DoFs and progressing on to other helicopter components, we return to the centre-spring model for a further examination of its merits as a general approximation.