King’s law

From Equation (3.2) it follows that in steady state the thermal power exchanged between the hot wire and the fluid stream is equal to the electrical power, W, dissipated in the wire by the Joule effect:

W = I2 Rw = E 2/Rw

where I is the electric current and E is the potential difference across the hot wire. From Equation (3.5), King’s law [1] (Equation 3.6) is obtained, linking the speed, U, to the fourth power of E:

IіRw = R = (a+ b4U){Tw -Ta) King’s law (3.6)

Vw

that taking into account Equation (3.1) becomes:

Equation (3.7) is a relationship between three variables: stream speed, U, electric current, I, and wire resistance, Rw. If the stream speed has to be related to a single variable (current or electrical resistance or potential difference) there are two possible alternatives:

■ holding the electrical resistance (or temperature) of the wire constant and allowing fluctuations of the electrical current with speed (constant temperature anemometer, CTA);

■ holding the electrical current constant: in this way the change in velocity causes a temperature change of the wire and hence of its electrical resistance (constant current anemometer, CCA).

Operating at constant resistance, differentiating Equation (3.7) yields:

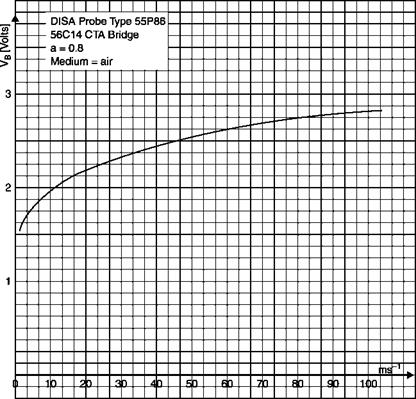
From Equation (3.8) it follows that (Figure 3.7):

■ the potential difference across the wire increases with increasing speed;

■ the instrument sensitivity decreases with increasing speed.

Operating at constant current the temperature of the wire is free to vary with speed; differentiating Equation (3.7) yields:

1 f3E) =- (Rw – Ra)Ra a(Tw – Ta)

EdUE 2 U + 2 U + abU

Calibration curve of a CTA

From Equation (3.9) it follows that (Figure 3.8):

■ the potential difference across the wire decreases with increasing speed;

■ as for the CTA, the sensitivity decreases with increasing speed.

A final consideration must be made on the effects of free convection that is taken into account by the term “a” in Equations (3.5), (3.6) and (3.7).

The fact that there is a small but finite convective velocity near the wire makes measurement of very low flow speed random. Furthermore, the value of free convection depends on the orientation of the wire (horizontal or vertical), so calibration and measurement have to be made in the same position. In practice, the King’s law, as mentioned earlier, has a lower limit of validity at stream speeds of the order of 5 cm/s.

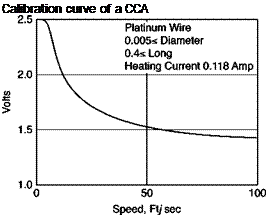Figure 3.8