Line (point) Vortex

A line vortex is a string of rotating particles. In a line vortex, a chain of fluid particles are spinning about their common axis and carrying around with them a swirl of fluid particles which flow around in circles. A cross-section of such a string of particles and the associated flow show a spinning point, outside of which the flow streamlines are concentric circles, as shown in Figure 5.8.

Vortices can commonly be encountered in nature. The difference between a real (actual) vortex and theoretical vortex is that, the real vortex has a core of fluid which rotates like a solid, although the associated swirl outside is the same as the flow outside the point vortex. The streamlines associated with a line vortex are circular, and therefore, the particle velocity at any point must be only tangential.

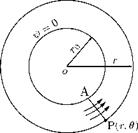
Stream function of a vortex can easily be obtained as follows. Consider a vortex of strength Г, at the origin of a polar coordinate system, as shown in Figure 5.9.

Let P(r, в) be a general point and velocity at P is always normal to OP (tangential). The radial velocity at any point P is zero, that is:

1 df г ~дв

since in polar coordinates, the radial velocity qr and tangential velocity qe, in terms of stream function f are: 1

For qr = 0, the stream function ф should be a function of r only. The tangential velocity at any point P [1] is:

_ Г _ дф 2 nr dr

Therefore,

Integrating along a convenient boundary, such as from A to P in Figure 5.9, from radius r0 (radius of streamline, ф = 0) to P(r, в), we get the stream function as:

— ln r _2n

that is:

This is the stream function for a vortex, and the circulation Г of a flow is positive when it is counter­clockwise.[3]

We know that the streamlines of a line vortex are concentric circles. Therefore, the equipotential lines (which are always orthogonal to the streamlines) must be radial lines emanating from the center of the vortex. Also, for a vortex, the normal component of velocity qn = 0. Therefore, the potential function ф must be a function of в only. Thus:

1 аф _ Г r de 2nr

Therefore:

Г

аф = — ав.

2n

Integrating this, we get:

Г

ф = — в + constant. 2n

By assigning ф = 0 at в = 0, we obtain:

This is the potential function for a vortex.

Also, we know that the stream function for a source [1] is:

where m is the strength of the source.

Comparing the stream functions of a vortex and a source, we see that the streamlines of the source (the radial lines emanating from a point) and the streamlines of the vortex (the concentric circles) are orthogonal.