Circular Vortex

A circular vortex is that with the shape of its cross-section normal to its axis of rotation as circular. For example a single cylindrical vortex tube, whose cross-section is a circle of radius ‘a’, surrounded by unbounded fluid, as shown in Figure 5.46 is a circular vortex.

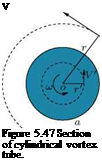
The section of this cylindrical vortex by the plane of motion is a circle, as shown in Figure 5.47.

Let us assume that the vorticity Z over the area of the circle is a constant. Outside the circle the vorticity is zero. Consider concentric circles of radii r’ and r, where r’ < a < r, as shown in Figure 5.47. Let the tangential speeds of the fluid motion on the circles of radii r’ and r are V and V, respectively.

We know that “the circulation in a closed circuit is the line integral of the tangential component of the velocity taken round the circuit in the sense in which the arc length (elemental length along the circuit) increases.” Thus, the circulation around the circles of radii r and r’, respectively, are:

V ds and V’ ds,

where ds is an elemental arc length. Also, V and V’ are constants. Therefore:

V ds = о)лаг, r >

V’ ds = ожг’г, r < a

where ю is the angular velocity. Thus:

2nrV

V

2nr’V’

V

when Г = r = a we have V’ = V = | аю so that the velocity is continuous as we pass through the circle.

From the above discussions, it can be inferred that the existence of a vortex implies the co-existence of certain distribution of velocity field. This velocity field which co-exists with the vortex is known as the induced velocity field and the velocity at any point of it is called the induced velocity. It is important to note that it is customary to refer to the velocity at a point in the field as the velocity induced by the vortex, but it is merely a convenient abbreviation of the complete statement that were the vortex to be alone in the otherwise undisturbed field the velocity at the point would have the value in question. In other words, when several vortices are present in the field, each will contribute to the velocity at a point.

For circular vortex the induced velocity at the extremity of any radius vector r joining the center of the vortex to a point of the fluid external to the vortex is of magnitude inversely proportional to r and is perpendicular to r. Thus the induced velocity tends to zero at great distances. The fluid inside the vortex will have velocity of magnitude proportional to r and therefore the fluid composing the vortex moves like a rigid body rotating about the center O with angular velocity ю = | f. The velocity at the center is zero. That is:

“a circular vortex induces zero velocity at its center."

Thus, a circular vortex alone in the otherwise undisturbed fluid will not tend to move.

The velocities at the extremities of oppositely directed radii are of the same magnitude but of opposite sense so that the mean velocity of the fluid within the vortex is zero. Thus, if a circular vortex of small radius be “placed” at a point in a flow field where the velocity is u, the mean velocity at its center will still be u and the fluid composing the vortex will move with velocity u. That is, the vortex will move with the stream carrying its vorticity with it.

Naturally occurring tropical cyclone, hurricane and typhoon which attains a diameter of from 150 to 800 kilometers, and travels at a speed seldom exceeding 25 kilometer per hour are circular vortices on a large scale. Within the area the wind can reach hurricane force, while there is a central region termed “the eye of the storm,” of diameter about 15-30 kilometers where conditions may be completely calm.