Estimate of the Efficiency and the Thrust Recovery of PAR Based on a Reentrant Jet Scheme

At the beginning, it is worthwhile to clarify the procedure for determin­ing PAR efficiency envelopes by using simple examples. First, we adopt the scheme of PAR flow with a reentrant jet (Fig. 8.1). We assume the potential character of the flow which enables us to use, in particular, the theory of jets in an ideal fluid [138]. We suppose additionally that the pressure on the boundaries of free jets is equal to the atmospheric pressure (zero perturbed pressure) and that the velocity is equal to f/j. We can write the PAR efficiency if par as

Kpar = (8.124)

where Cy = 2Ry/pU? S is the lift coefficient, S is the reference area of the wing, and Ct = 2T/pU? S is the thrust coefficient. Note that both coefficients are based upon the dynamic pressure head of the jet and the reference area of the wing. We introduce thrust recovery fraction TT

T — Rx Rx Cx, .

Tr = —= 1 –f = 1 – cp (8-125)

where Cx = 2Rx/pU? S is the drag coefficient. Applying horizontal projection of the momentum theorem to the surface bounded by flow cross sections at the nozzle of the PAR engine, the incoming jet (/-/), the reentrant jet (II – II) and the escaping jet (111-І 11), we deduce the relationships between the thrust (drag) forces and the width of the participating jets. For example, applying the above mentioned theorem to the flow cross sections at the nozzle and (1-І) accounting for the direction of the outward normal, we obtain

T cos#j = pU?5jC0, (8.126)

where C0 is the wing’s root chord. Hence, by using the definition for the thrust coefficient,

Ct cos<9j =25y (8.127)

It follows from (8.127) that, within the assumption of the potential flow theory, the horizontal projection of the thrust coefficient is equal to the dou­bled magnitude of the relative (i. e., expressed as a fraction of chord) width of the jet in front of the wing 5j.[42] Later on, for simplification of presentation, cos0j is omitted, so that Ct will be understood as the horizontal component of the same coefficient. Generally speaking, coefficients Cy and Ct depend on quite a number of factors (e. g., the flap deflection angle, the angle of pitch, the geometry of the wing, particularly on the form of its lower surface, the magnitude and distribution of the effective gaps under endplates in direction of the chord, etc.). However, for the moment, to illustrate the method of trac­ing PAR efficiency envelopes, an example of a wing with no lateral leakage at zero incidence 9 = 0 and with a flap set at an effective gap 5f is considered.[43] In this case, it follows from the previously derived formulas that

Cy = l-sj, (8.128)

Cx = h(l-8f)2, (8.129)

where Sf = 5f/h, and h is the ground clearance at the trailing edge. Note that for zero incidence, the lift and the ideal pressure drag acting upon the wing are due only to the deflected flap. The suction force is not accounted for in the reentrant jet model.

Taking into account (8.124), (8.125), (8.127), and (8.128), we write the PAR efficiency and the thrust recovery fraction as

Cy(5f)h Cy(Sf) 1 – S2{

KpARh = – cr = ^- = ^l-‘

Cx(6uh) Cx(St, h) (1-Jf)2

Ct 25) 25)

where <5) = Sj/h.

Excluding the parameter 2Sj from (8.130) and (8.131), we obtain the relationship of the PAR efficiency to the thrust recovery fraction for given magnitudes of Sf.

On the other hand, excluding the parameter <5f from the same equations results in the expression of KpARh as a function of Tr for a given <5j. In fact, it follows from (8.131) that

St = 1 – /2<5j(l-Tr). Substituting (8.133) in (8.130),

Formulas (8.132) and (8.134) define sets of curves of constant values Sf = const, and Sj = const. Both the formulas and the corresponding diagrams show how to meet the required PAR efficiency and thrust recovery fraction magnitudes by an appropriate setting of the trailing edge gap for a given thrust coefficient or by securing the required thrust coefficient for a given trailing edge gap. Note, that for a thrust recovery fraction Tr ranging from zero to unity, the domain of variation of the PAR efficiency is bounded

both from below and from above. The lower bound can be determined from formula (8.132) for Sj 0 as

(KPARh)min = l-TT. (8.135)

As stated earlier, the equation of the upper bound of the PAR efficiency diagram can be obtained by applying the momentum theorem for a control surface, including cross sections 1-І, 11-І I and III-III. As a result,

pUf <5j C0 – pUf Suj C0 cos /?j – pUf Sf C0 = RX, (8.136)

or, in nondimensional form,

25j – 2Juj • cos/3j – 26{ = Cx, 2Jj = Ct. (8.137)

Accounting for the mass conservation condition

(8.138)

and substituting a concrete expression for Cx in the case under consideration by (8.129), we obtain the following expression:

(-^•PAR * ^)max —

A certain magnitude of the reentrant jet orientation angle /?j corresponds to each pair of parameters (5j, Sf). Therefore, it is possible to find a magnitude of this angle, for which the coefficient of required thrust (or the required width of the incoming jet) would be minimal. This takes place at fy = тг, resulting in the following equation for the upper boundary of the PAR efficiency diagrams:

where

= 2^jm, n = 2ft + (1- /fl2 = 1(1 – ^f)2- (8.141)

As can be shown from (8.128) and (8.141), for the optimal blowing case, parameter 5{ is related to the thrust recovery fraction Tr in the following way:

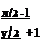It follows from (8.142) that in the case under consideration, nonnegative values of Гг are reached if

(8.143)

The maximum PAR efficiency, corresponding to optimal blowing, can be expressed by the formula

(■КРАЯ ‘ h)max = ) • (8.144)

Combining (8.142) and (8.144), we can derive a simple relationship between the efficiency of power augmentation and the thrust recovery fraction for optimal organization of blowing:

(^PAR ‘ h)max = л/2(1 ~ Tr). (8.145)

Note that for a fixed magnitude of the thrust coefficient Ct and a varying flap setting 5f, the efficiency of power augmentation varies between its minimal and maximal values, and the thrust recovery fraction ranges between its lower and upper bounds

(8.146)

On the other hand, for a fixed trailing edge setting Sf and varying thrust coefficient Ct, the thrust recovery fraction changes in the range

1 A 2

maxjo, 1 — 2^-—j < Tr < 1. (8.147)

Based on the preceding analysis, we can conclude that the realization of the optimal blowing regime associated with maximum PAR efficiency im­plies “tuning” of parameters <5j (or Ct) and in accordance with equation (8.141). If, for a given setting of the trailing edge gap, the incoming jet is wider than the optimal width, it spills over the leading edge. In this case, as indicated in [155], this redundant part of the jet does not participate in the PAR mechanism of lift. On the other hand, if the jet coming to the wing is thinner than required by the upper bound, the blowing may become com­pletely ineffective owing to insufficient pressure recovery at the entrance of the channel flow. It is remarkable that theoretical analysis of the local flow around the leading edge, based on a reentrant jet scheme (see paragraph 1.1.1), shows that the corresponding solution exists if

2<5fj > 25f + + ^o)2)

the upper bound of the domain of existence of the solution, can be shown to coincide with the equation for the curve of maximum PAR efficiency. For the simple case under consideration, v* = — <Sf, hi — 1, wherefrom it can

be seen that the equality

2*jmin=25f + i(l-£-f)2

coincides with requirement (8.141). The PAR efficiency envelope for the sim­ple case considered above (i. e., zero gaps under tips of the endplates, a flat

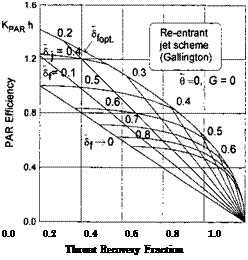Ct

Fig. 8.12. Envelopes of PAR efficiency based on a reentrant jet scheme.

lower foil surface and a zero pitch angle) is presented in Fig. 8.12. In some cases, for reasons of practical use of the PAR efficiency diagrams, it is con­venient to trace these diagrams, using a set of constant thrust coefficients Ct = const., Sf = var. rather than a set of constant incoming jet thicknesses (<5j = const., Sf = var.).