THE STATIC MARGIN

The distance between the centre of gravity and the neutral point is termed the static margin of the aircraft. It gives a very useful standard of comparison of one aircraft with another, since if they have similar static margins they will have similar static stability. The larger the margin, the greater the stability. This concept also brings into emphasis that a shift of the centre of gravity of any model aircraft can change the stability margin. By this very simple means a dangerously unstable model can be made stable, or an over-stable one made more sensitive and responsive. Stability is thus almost entirely under the control of the model flier and can be varied, within limits, by the addition or subtraction of ballast at nose or tail. Any such change of ballast of course will require a new trim. setting.

12.14 LOCATION OF THE NEUTRAL POINT

As just mentioned, the model flier does not actually need to know where the neutral point of his aircraft is, because stability can so easily be adjusted by careful use of ballast

Fig 12.11 Stable layouts: C. G. ahead of N. P.

/

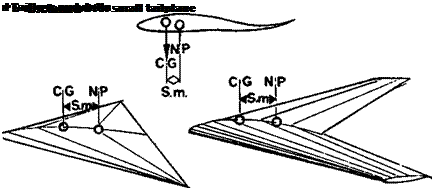However, it is useful and interesting to know how the neutral point may be found if a new model is being designed or if two models are being compared.

The most important determinants of neutral point position are the mainplane and the tail or foreplane. Any lifting surface ahead of the centre of gravity will naturally tend to move the neutral point forward and so* is destabilising. This applies to canards. The foreplane causes the neutral point to lie ahead of the mainplane’s aerodynamic centre, so a centre of gravity position like that of Figure 12.4 is unstable. For stability, the canard must have the c. g. forward as shown in Figure 12.11c. Thus, the foreplane of a stable canard carries, for trim, not only the camber induced pitching load, but an additional load caused by the forward c. g. Any surface behind the centre of gravity, such as a tailplane, has a stabilising effect, since it brings the neutral point aft. Unfortunately, the efficiency of the tailplane is adversely affected by the wing, especially if it lies in the wing wake or comes into the wake at some angles of attack. Despite this, a useful measure of the tailplane’s stabilising effect may be obtained by working out the stabiliser volume coefficient

Fig. 12.12 Unstable layouts C. G. behind N. P.

a Orthodox layout

The formula relates the tail volume to the wing and fuselage length:

у _ Ss x Ls Sw x c

Here, Vs is the tail volume coefficient, Ss and Sw are tail and wing areas respectively, Ls is the distance of the tailplane’s quarter chord point from the wing’s aerodynamic centre (allowing for any sweep of either surface), and c is the mean wing chord (Sw/Span). Strictly, the coefficient so found should be reduced by some factor to allow for loss of tail efficiency. A high-mounted T tail may be as much as 90% efficient, a low tailplane behind a fattish fuselage, in the wake from the wing, may be only 50% efficient. Some guesswork is involved in making such estimates. For a canard the same formula may be used to assess the de-stabilising effect of the forewing.

Various ways are used to calculate the position of the neutral point, using the wing and tail alone and ignoring any other effects. A way of doing this without calculation is suggested in Figure 12.13. Such crude methods are of course only approximate. A method of more exact calculation appears in Appendix 1.