The Induced Lift
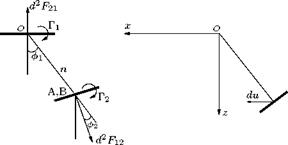
Let us consider two vortex elements with circulations Г1 and Г2, as shown in Figure 8.20. The velocity induced at ds2 by ds1 along Ox is:
Г 1ds1
du = ——- — cos в cos Ф1.
4nr2
This induced velocity is against the wind. This induces a lift in the element ds2, given by:
2 p Г1Г2
d F12 = рГ2ds2du = —- — ds1 ds2 cos в cos ■
4nr2
|
Figure 8.20 Two vortex elements. |
The velocity induced at ds1 by ds2 is with the wind, and the induced lift is:
2 p Гі Г2
d F21 = —— 7T – ds1 ds2 cos в cos ф2
4nr[14] [15]
Resolving along n, the projection of the line joining the elements on a plane normal to the wind, we get:
d2F12 cos ф2 — d2F21 cos фі = 0.
Resolving perpendicular to n, we get:
Лъ • , ,2 V ■ . P Г1Г2 ds1 ds2 „ . ,, ,4
d2 F21 sin Ф1 — d F12 sin Ф2 = ——- —— cos в sin (Ф1 — Ф2).
4nr2
This vanishes when фі = ф2 and is small in general.