The Aerodynamic Efficiency of a Wing in the Extreme Ground Effect

9.1 Optimal Wing-in-Ground Effect

The theory of a wing in the extreme ground effect, as developed herein, enables us to formulate a number of extremal problems, which are of both theoretical and practical interest.

We consider the conditions of the minimality of the induced drag of a lifting system in the extreme ground effect in Munk’s sense [159]. We as­sume that a thin vortex wake extending behind the trailing edge represents a cylindrical surface with a generatrix parallel to an unperturbed underlying boundary. This assumption permits us to assume that, at a sufficient distance downstream from the lifting system, the flow is close to two-dimensional in the plane normal to the direction of motion (a Trefftz plane). It is known that the induced drag coefficient can be expressed by the integral over the whole Trefftz plane St of the kinetic energy of a fluid per unit length in the direction of motion, see Ashley and Landahl [161] and Barrows and Widnall [160]:

CXi =Y2Л (9.1)

In formula (9.1), all quantities were rendered nondimensional by using some characteristic (cruise) speed U0 and the length of the root chord C0. The differential d5x represents a differentially small area element in the Trefftz plane, related to the square of the root chord. Quantities Л and l represent, respectively, the aspect ratio and the relative span of the lifting surface, (p is the velocity potential of the perturbed motion of the fluid.

Using Green’s theorem, we can transform integral (9.1) into a contour integral:

where Cjj is the contour in the Trefftz plane that incorporates a contour Cl that encloses the wake and a contour C2 that coincides with the line of intersection of the ground and the Trefftz plane. Taking into account the tangency condition on the ground, we can transform (9.2) into an alternative form

K. V. Rozhdestvensky, Aerodynamics of a Lifting System in Extreme Ground Effect © Springer-Verlag Berlin Heidelberg 2000

C-‘ – P І Vtn a = P il’ (9’3)

where C is the contour of the wake passed in one direction. Within the as­sumptions of linear theory, the expression for the lift coefficient for a wing of small aspect ratio with a curvilinear lateral axis can be written in the form

C, = ^ /i<I’)c°s<n,!’,dS = f Д(:aT~llr)cos("’!/,’:ls

2Л r 2X f

= JJ-V+ ~<P-)<x>s(n, y)dl = – jj J^r(l)cos(n, y)dS, (9.4)

where n is a normal to a curvilinear cut that represents the wake in the Trefftz plane. To determine the conditions of the minimum of the induced drag for a given magnitude of the lift coefficient, we find a minimum of the function

V = CXi-*Cy, (9.5)

where A* is the variational Lagrange multiplier. Then, in accordance with variational calculus, a variation of the function V must be equal to zero, that is,

2A f

SCXi = -p VtpV6<pdST. (9.6)

St

Turning to contour integration with the help of Green’s formula, we obtain

SC“ = ж fSrllnil’ <9’7>

c

— A* cos(n, y)

Taking into account (9.7), we obtain

For an arbitrary variation of the equality (9.8) is possible only if

= A*cos(n, y). (9.9)

It can be seen from (9.9) that A* represents the vertical downwash w0 in the middle of the wake. Thus, the expression (9.9) can be rewritten as

dip

— =vn = w0 cos(n, г/), (9.10)

which corresponds to the following theorem (Munk [159]): The induced drag of a wing is minimum if the normal component of the induced
downwash at each point is proportional to the angle of inclination of the lifting element at this point. Taking into account (9.10), the expressions for the coefficients of the lift and the induced drag of an optimal wing take the form

– 12	a II "3 9-І £ cs |cs	rl/2 Г(г) dz, – it 2	(9.11)
2A /	’ 2A	fl/2 x x	(9.12)
Cy = lJ j	r(l)cos(n, y)dl = – p	r(z)dz. J—1/2

Using Prandtl’s representation of the relationship between the lift and the induced drag coefficients, we obtain

where

The product A/і = Ae is called the effective aspect ratio of the wing and can be used as a measure of the aerodynamic efficiency of the lifting system.

h*(x, z),

dh* Их’

dh* „ -5- < 1, dz

Now, we can find the form of the optimality condition for a wing in close proximity to the ground. Recalling that in the asymptotic theory, discussed herein, both the gap and its chordwise derivatives are assumed small

h*(0,z)

and by using the formula for the determination of induced downwash at points of the wake in steady motion, we obtain the following form of the optimality condition for a laterally curvilinear wing in the extreme ground effect:

where h*(0, z) is the distribution of the distances of points of the trailing edge from the ground and Г = T(z) is the distribution of the circulation along the trailing edge of the wing. Integrating (9.16) taking into account that the loading must vanish at the tips of the wing,

г-=”"/-‘/жЛ2′ (917)

The constant C was determined by using the requirement that the circulation should vanish at the extremities of the wake (wing).

If the lateral curvature of the wing’s surface is negligible, Munk’s theorem is reduced to the requirement that the downwash should be constant span – wise. In the limit of vanishing clearances h and taking into account (9.10), the requirement of optimality of a wing in the extreme ground effect can be reduced to

Integrating (9.19) and taking into account that the tip loading should be equal to zero, i. e., ^(0, ±A/2) = 0, we obtain

iph = ^K{z2~x2)- (9-20)

Thus, the optimal wing in the extreme ground effect has a parabolic spanwise distribution of circulation. This conclusion reveals a distinction of the aerodynamics of the extreme ground effect from that in an unbounded fluid, where the optimal wing has an elliptic loading distribution spanwise. It is also compatible with the results of de Haller [134], who obtained an exact solution for an optimal wing in the Trefftz plane in terms of an infinite series of elliptic functions and demonstrated by calculations that, when the clearances diminish from infinity to zero, the optimal loading distribution changes from elliptic to parabolic. Returning to the solutions derived in paragraph 3.5, we can see that a semielliptic wing is optimal for any aspect ratio and that a flat wing of small aspect ratio in the extreme ground effect is optimal independently of its planform. Similar conclusions follow from the theory of a lifting line(s) in the extreme ground effect, set forth in section 10, where parabolic spanwise loading also furnishes the minimal induced drag for a given lift.

Using the results of linear theory, stated in paragraph 3.4, we can study the requirements for the optimality of a rectangular wing of an arbi­trary aspect ratio. In a sufficiently general case, the spanwise distribution of the circulation at the trailing edge of a rectangular wing for h —> 0 can be written as follows:

oo ^

Vli (0; z) — ^2 an cosqnz, qn = -(2n + l). (9.21)

71=0

Comparing expressions (9.20) and (9.21), we can determine the coefficients subject to the optimality condition:

Due to the fact that the coefficients an reflect the specifics of a concrete problem, condition (9.22) enables us to find the optimal spanwise distribution of the different parameters, such as aerodynamic twisting, jet flap momentum distribution, etc.

For example, we can find such a distribution of the angle of pitch for which a rectangular wing of arbitrary aspect ratio has a minimum induced drag. From the solution of the corresponding flow problem for an arbitrary spanwise distribution of the angle of pitch 0(z) = 9o0(z), presented in paragraph 3.4, it follows that at a point on the trailing edge

У’іДО, z) = –Г "T tanh qn tanh cos qnz, (9.23)

h n=o Яп 1

where

2 /^/2

0n = – r 0(z) cos qnz dz.

л J-X/2

The coefficients вп in accordance with (9.22) can be derived from the equation

Consequently,

Therefrom, the optimal spanwise distribution of 9{z) for a rectangular wing (aerodynamic twist) of arbitrary aspect ratio A is described by the following equation:

<a ( – 4u>° ( l)n cos Qnz

~ °pt 2 A0O ^ qn tanh qn tanh(g„/2)’

For a wing of small aspect ratio A -> 0 (qn ->• oo), this expression yields

and therefore, no aerodynamic twisting is required for the optimiza­tion of a rectangular wing of small aspect ratio.

For a wing of large aspect ratio Л oo (qn 0),

It follows from (9.25) that an optimal rectangular wing of large aspect ratio in the extreme ground effect should have a parabolic distri­bution of the angle of pitch. It can be shown that a noticeable gain in the

lift-to-drag ratio can be achieved only for wings of sufficiently large aspect ratios.

In another example, we find the optimal distribution of the jet mo­mentum along the trailing edge of a rectangular wing with a jet flap. Based on the results obtained in paragraph 6.2 of section 6, at points of the trailing edge of a jet-flapped rectangular wing-in-ground effect,

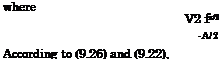an = —r – [ Jc^z) cos qnzdz. л J-A/2 v

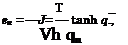4w0(—1)"

Vhql ’ from which

4u;0(—l)ra

a” Arqltanh qn ’

so that the optimal distribution of the jet velocity distribution along the trailing edge is given by the following expression:

Setting the aspect ratio to infinity, we derive a parabolic distribution of the jet velocity in the form

For a wing of small aspect ratio,

fc; _ 4w0 (-l)ncos qnz _ 4w0

V 2 – At ^ ql ~ At

Bearing in mind that the derivative of S(z) can be summed up in a closed form (see Gradshtein and Ryzhik[147], p. 52),

and integrating (9.28), we can express the right-hand side of (9.27) by the Lobachevsky function L(u) (see Gradshtein and Ryzhik [147]),

This formula describes an optimal law of ejection of air along the trailing edge of a rectangular wing with a jet flap.