Calculation of the Downwash Velocity

Consider first the velocity induced at P(x, y, 0) by a vortex MM’, shown in Figure 8.22, parallel to the span (lifting line) and the trailing vortices which spring from it. Let Q(x’, y’, 0) be a point on the vortex MM’. The circulation at Q is then у1 (x’, y’) dx’ and from Q there trails a vortex of circulation:

дуіС! У) , ,

————– dy dx.

dy

The downwash velocity induced by the trailing vortex caused by MM’ (see Chapter 5) at point P is:

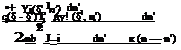Thus from Equation (8.69) we get:

,2 (§ §)2 , ( /) 2

к = ——— — + (n — n) .

AT

Note that, if §’ = § Equation (8.72) reduces to its first term and if we put y W, n) = Yo (§0 /(1 — n’2)- the elliptic distribution across the span, we get:

Yo (§’) + c(§ — §,)Yo(§,) f+l_________ n’ dn’______

2b 2nb2 J—i k(n — n’) /(1 — n’2)

Substituting Equation (8.74) into Equation (8.7i) we get the downwash velocity. The induced drag is given by:

which includes the suction force at the leading edge and hence the leading edge should not be rounded.

The integrals in Equation (8.74) are elliptic type and cannot be evaluated in terms of elementary functions. Blenk therefore adopted an ingenious method of approximation, even though it is lengthy. However, this approximation is valid only to the middle part of the wing so that the end effects are uncertain. The approximation is better suited for larger aspect ratios. The method leads to replacing Equation (8.74) by:

where the coefficients Ai, Bi, Ci, Di and Ei are functions of n which depend on the particular case among the four wing shapes considered. For the rectangular wing moving in the plane of symmetry:

The downwash may be calculated from Equation (8.7i) with the aid of Equation (8.75)

To determine the profile of the section at distance y from the center, let us assume the relative wind to blow along the x-axis. Since we consider the perturbations in the freestream to be small and the air must flow along the profile, we have:

dz w

дХ = V’

Therefore:

і г. .

z = — / w(x, y) dx. V

Comparison of this result with the theory of the lifting line gives the following mean additions to the incidence and curvature for the rectangular wing:

C і C

Aa = 0.059 A – = 0.056

M R b

In the case of sweep-back wing, the mean increase of incidence, according to Blenk, should be 1’6 в (4 — М) percent of the absolute incidence without sweep-back.