Rectangular Aerofoil

Let us assume that the load distribution is given for the rectangular planform of Figure 8.21(a). Now the task is to find the profiles at different sections.

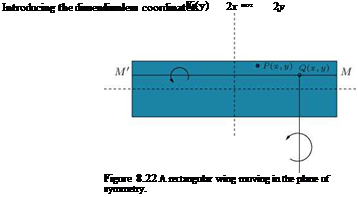
Let us assume the profile to be thin so that the whole aerofoil may be considered to lie in the xy plane, as shown in Figure 8.22.

Let Yi (x, y) be the circulation per unit length of chord at the point (x, y, 0) so that the circulation round the profile at distance y from the plane of symmetry is:

[where c is the chord and b is the span (note that here b is taken as the span, instead of 2b, for convenience)] we get the circulation as:

nfa) = J Y(§, n) d§. (8.66)

For y(§, n), let us choose the following elliptic distribution over the span:

Y(§, n) = У0Й) V7! – П2

and for у0(§), let us consider the following three different functions:

where a0, b0, c0 are arbitrary constants. Note that Equation (8.67a) is the distribution for a thin flat aerofoil in two-dimensional motion. The most general distribution considered here will then be of the form: