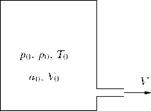Discharge from a Reservoir

Consider a reservoir as shown in Figure 9.2, containing air at high pressure p0. Let the density, temperature, speed of sound and velocity of air be p0, 70, a0 and V0, respectively.

Because of the large volume of the reservoir, the velocity of air inside is V0 = 0. Let the high pressure air be discharged to ambient atmosphere at pressure pa and velocity V = 0, through an opening as shown in Figure 9.2. Now the velocity V at the opening, with which the air is discharged, can be obtained by substituting V1 = 0, p1 = p0, P1 = P0 and p2 = pa into Equation (9.19) as:

 

V

 

(9.21)

 

Figure 9.2 Discharge of high pressure air through a small opening.

 

For discharge into vacuum, that is, if pa = 0, Equation (9.21) results in the maximum velocity:

Vmax is the limiting velocity that may be achieved by expanding a gas at any given stagnation condition into vacuum. For air at TO = 288 K, Vmax = 760.7 m/s = 2.236 a0. This is the maximum velocity that can be obtained by discharge into vacuum in a frictionless flow. From Equation (9.22), we can see that Vmax is independent of reservoir pressure but it depends only on the reservoir temperature. For incompressible flow, by Bernoulli’s equation:

Therefore:

In this relation p is replaced by p0, because p is constant for incompressible flow. Combining Equations (9.22) and (9.23a), we get:

For air, with y = 1.4:

Vmax (comp.) ^ 1.9 Fmax (incomp.).

That is, the error involved in treating air as an incompressible medium is 90%.

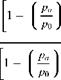For the case when the flow is not into vacuum, pa/p0 = 0 and Equations (9.21) and (9.23) may be expressed by dividing them by a0 as:

In the course of discussion in this section, we came across three speeds namely, Vmax, a0 and V* (= a*) repeatedly. These three speeds serve as standard reference speeds for gas dynamic study. We know that for adiabatic flow of a perfect gas, the velocity can be expressed as:

v = ^2cp (T0 – t) = yY-IRTw),

where T0 is the stagnation temperature. Since negative temperatures on absolute scales are not attainable, it is evident from the above equation that there is a maximum velocity corresponding to a given stagnation temperature. This maximum velocity, which is often used for reference purpose, is given by:

Another useful reference velocity is the speed of sound at the stagnation temperature, given by:

ao = J yRT0-

Yet another convenient reference velocity is the critical speed V*, that is, velocity at Mach Number unity, or:

V* = a*.

This may also be written as:

R(T0 – T*) = у/ЇВТ*.

Y – 1

This results in:

T * _ 2

To Y + 1

Therefore, in terms of stagnation temperature, the critical speed becomes:

From this equation, we may get the following relations between the three reference velocities (with

Y = 1.4):