A3 ATMOSPHERIC DISTURBANCE MODELS

Turbulence Models

The movement of the air in the atmosphere creates turbulence. The velocity of air may vary in a random fashion in both space and time. The two popular mathematical model forms that explain the random behavior of turbulence are the Dryden and the von Karman model.

The power spectral density for the turbulence velocity given by the Dryden model (Example 6.3) is as follows:

where V = v = 2p, V is the spatial frequency, v is the circular or temporal fre­quency, V is the aircraft speed, l is the wavelength, Lu, Lv, Lw are the scale of the turbulence and su, sv, sw are the turbulence intensities. The subscripts u, v, w represent the turbulence velocity components.

von Karman’s spectral representation of turbulence is given by [1]

The spectral representation given by von Karman is widely used to simulate turbu­lence effects.

Longitudinal Model with Gust

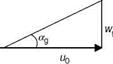
The rigid body airplane equations of motion were discussed in Chapter 3 and the aerodynamic models for force and moment coefficients, in terms of Taylor’s series, were discussed in Chapter 4. Here, we present the longitudinal aerodynamic model assuming one-dimensional gust (only wg, i. e., up and down gust), since wg is mainly responsible for normal accelerations. The upward gust is assumed positive as it produces positive increment in the AOA (Figure A6).

FIGURE A6 Upward gust.

The AOA and pitch rate due to gust are given by [6]

Wg

°g =— and qg = —° g

U0

The aerodynamic model equations for vertical force and pitching moment coefficient can therefore be expressed as

Cz = Cz°° + °g) + Czq(q – °g) 2^ + Czdpe

c c

Cm — Cm°(° “b °g) “b Cfflq(q °g) “h Cm°° “b ^°g) “h Ст§уе

2U0 2U0 e