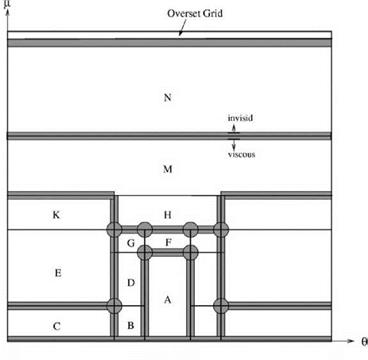
Selection of Time Step

The time step used in the computation is selected to meet the stability and accuracy requirements of the subdomain with the smallest size mesh. In the computation domain, the governing partial differential equations have variable coefficients. It is impractical to perform numerical stability analysis for equations with variable coef­ficients. Here, the frozen coefficient approximation is invoked. Under this approxi­mation, the coefficients are treated as constants. To further simplify the analysis, the viscous terms that are diffusive in nature will be neglected. In the д – в plane, the linearized governing equations are Eq. (5.61), which may be written in the following form:

-7t Wall Boundary 7Г Figure 15.29. Distribution of extra artificial selective damping (shown shaded).

where

p"		иДх + V py	PPx	PPy	0
и	, a =	0	иДх + V py	0	Px/p
v		0	0	U^x + V^y	PyfP
P-		0	Y pPx	Y PPy	Upx + VPy

u

x

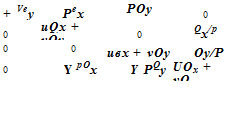
В

where subscripts x and y indicate partial derivatives. u, v, p, and p are local values. Coefficient matrices A and В are to be regarded as constants in the analysis.

Numerical stability analysis follows the procedure of Section 5.3. For this pur­pose, the Fourier-Laplace transform is applied to Eq. (15.49) locally. The Fourier- Laplace transform in the p – O plane is defined by

TO

U(а, в,а>) = ^2^3 j j j u(p, O, t) e—l(ap+eo—mt) dp de dt.

— TO

The Fourier-Laplace transform of Eq. (15.49) is

0 ю — (a u + P v) 0 — a/p

0 0 ю — (a u + P v) —P /p

0 — y pa — – pP ю — (au + j3v) where a = a/лх + вд p = aQx + вQy. The eigenvalues of matrix К are as follows:

■1 = ■Ч = [ю — (au + p v)]

■3,4 = ю — (au + Pv) ± (yp/p)1/2(a2 + P2)1/2.

The dispersion relation that imposes the most stringent stability requirement is that of the acoustic wave Л4 = 0. On proceeding as in Section 5.3, it is straightforward to derive the following maximum time step formula should the 7-point stencil DRP scheme be used for the computation.

15.4.1.2 Starting Conditions

In the course of performing the airfoil tone simulations, it has been found that the following simple starting conditions have been very successful:

1

t = °, u = ^incoming, v = 0> Р = 1 P = -•

The uniform starting condition is applied throughout the entire computational domain. In order to comply with the no-slip boundary conditions on the airfoil surface, the boundary conditions on the airfoil surface, д = 0, is taken to be

Here, t is recommended to be five or six times of the tone period. The no-slip boundary conditions are attained at t = T.