Flow over a Wave-Shaped Wall 507404511

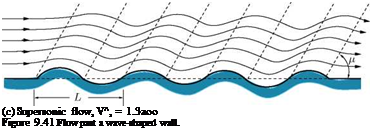
Consider a uniform flow of velocity Vx over a two-dimensional wave-shaped wall, as shown in Figure 9.41, with wavelength L and amplitude h.

Let the wall shape be defined by the equation:

zw = h sin(Xx). (9.201)

In Equation (9.201), subscript w stands for wall and X = 2л/L. Let us assume h ^ L, so that linear theory can be applied. By kinematic flow condition [Equation (9.68)], for z ^ 0, we have:

—- = —XXL – = hX cos (Xx). (9.202)

Lx, dx

Now, with this background, let us try to solve the governing equation for incompressible flow, compress­ible subsonic flow and supersonic flow.

9.25.1 Incompressible Flow

The governing equation for incompressible flow is the Laplace equation:

Fx + Фzz = 0.

This can be solved by expressing the potential function as:

Ф^, z) = F(x) G(z).

Solving by separation of variables, we get:

ф^, z) = — Vxhe Xz cos (Xx).

V

The potential function given by Equation (9.203) is only the perturbation potential. Obtaining the ex­pression for ф, given by Equation (9.203), is left as an exercise to the reader.

Using Equation (9.203), we can easily get the resultant velocity U and perturbation velocity w as:

U = + u = VOT ^ 1 + hke~lz sin (kx) j (9.204a)

w = V(x, hke^kz cos (kx). (9.204i>)