The Method of Stationary Phase

Consider evaluating the following integral in the limit X ^ ro.

b

1,X) = и, х)е"»<Ъ.

a

It was pointed out by Lord Kelvin (1887) that for large X the function еіЛФ(х) will oscillate wildly with almost complete cancellation. Significant contribution to the integral comes from small intervals of х where Ф(х) varies slowly. This happens when Ф'(х) = 0 or at the stationary points of Ф(х).

Suppose Ф(х) has only one stationary point in (a, b), say at х = с. That is

Ф/;, с)

Ф, х) = Ф, с) +—— 2C, х – c2 ) + •••• (B2)

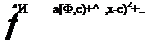Since the major contribution to (B1) comes around х = с, asymptotically the integral is given by

C+S

И, с)е1ХФ, с) еФ" ,c),x-c)2Dx.

с—S

It is possible to change the limits of integration to – ro to ro as the error of extend­ing 5 to ro is asymptotically small (nearly complete cancellation). On accounting for that Ф"(с) may be positive or negative, the integral of (B3) may be evaluated to give

b 1

lim И, х)еаФ, х)ёх – — 2 И, cyH(c)+sgn^"(c))f], (B4)

x->ro w X Ф, c)| w ()

a

where sgn( ) is the sign of ( ).