Thin Airfoils in Incompressible Flow

Considering first the symmetric problem for an airfoil at M = 0 of chord c we seek a solution of

<Pxz + Vzz = 0, (5-39)

subject to the boundary conditions that

<рг(х, 0±) = ±r ^ for 0 < x < c (5-40)

and that the disturbance velocities are continuous outside the airfoil and vanish for v/a:2 + z2 —» oo. Since we are dealing with the Laplace equa­tion in two dimensions, the most efficient approach is to employ complex variables. Let

Y = x + гг

V?(Y) = <p(x, z) + гф(х, z) (5-41)

dW, . . , ,

q = – j=: = u(x, z) — iw(x, z),

where q is the complex perturbation velocity vector made dimensionless through division by U„. In this way we assure that, provided Ф is analytic in Y, <p and Ф, as well as q, are solutions of the Laplace equation. Thus we may concentrate on finding a q(Y) that satisfies the proper boundary conditions. In the nonlifting case we seek a q(Y) that vanishes for | Y —> oo and takes the value

q(x, 0±) = u(x, 0±) — iw(x, 0±)

= u0(x) =F iw0(x), say, for 0 < x < c, (5-42)

where

w0(x) = T~ (5-43)

The imaginary part of q(Y) is thus discontinuous along the strip z = 0, 0 < x < c, with the jump given by the tangency condition (5-40). In order to find the pressure on the airfoil we need to know u0, because from (5-31)

Cp(x, 0) = —2<px(x, 0) = — 2w0. (5-44)

To this purpose we make use of Cauchy’s integral formula which states that given an analytic function /(Fx) in the complex plane Fx = X + iz, its value in the point = Y is given by the integral

/(F) – 2ш? с Y] – Y’ (5’45)

where C is any closed curve enclosing the point Fx = Y, provided /(Fx) is analytic everywhere inside C. We shall apply (5-45) with / = q and an integration path C selected as shown in Fig. 5-6.

The path was chosen so as not to enclose completely the slit along the real axis representing the airfoil because q is discontinuous, and hence nonanalytic, across the slit. Thus

In the limit of Ri —> oo the integral over Ci must vanish, since from the boundary conditions q(Yj) —> 0 for Yi —> oo. The integrals over the two paths C2 cancel; hence

m _L f q(Y i) dY і J_ f° А д(хг) dxx

qK > 2wiJc3 Yi – Y 2mJo xx — Y ’

where Aq is the difference in the value of q between the upper and lower sides of the slit. From (5-42) it follows that

Ag(xi) = q(xu 0+) — q(xu 0—) = —2гад0(ж1). (5-48)

Hence, upon inserting this into (5-47), we find that

which, together with (5-43), gives the desired solution in terms of the air­foil geometry. Separation of real and imaginary parts gives

In the limit of г —» 0+ the second integral will receive contributions only from the region around xx = x and is easily shown to yield w0 as it should. To obtain a meaningful limit for the first integral, we divide the region of integration into three parts as follows:

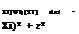
(5-52)

where 6 is a small quantity but is assumed to be much greater than z. We may, therefore, directly set z = 0 in the first and third integrals. In
the second one, we may, for small S, replace w0(xi) by w0(x) as a first approximation, whereupon the integrand becomes antisymmetric in ж — x and the integral hence vanishes. The integral (5-50) is therefore in the limit of z = 0 to be interpreted as a Cauchy principal value integral (as indicated by the symbol C):

Uo{x) = lf^(xiUxl! (5-53)

IT J0 x — Xi

which is therefore defined as

Turning now to the lifting case, we recall that и is antisymmetric in z, and w symmetric. Consequently, on the airfoil,

w{x, 0) = w0(x) = в ^ — a (5-55)

is the same top and bottom, and in (5-45) then

Дq = u(x, 0+) — u(x, 0—) = 2u0(x) = У(х), (5-56)

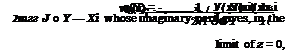where У(х) is the nondimensional local strength of the vortices distributed along the chord. Hence, (5-47) will yield the following integral formula

This is the integral equation of thin airfoil theory first considered by Glauert (1924). Instead of attacking (5-58) we will use analytical tech­niques similar to those used above to obtain directly a solution of the complex velocity q(Y). This solution will then, of course, also provide a solution of the singular integral equation (5-58). For a more general treatment of this kind we refer to the book by Muskhelishvili (1953).

Again, we shall start from Cauchy’s integral formula (5-45) but this time we instead choose

f(Y) = g(Y)h(Y), (5-59)

where h(Y) is an analytic function assumed regular outside the slit and sufficiently well behaved at infinity so that /(F) —> 0 for Y —> сю.

(5-66)

For the integral to converge, m and n cannot be smaller than —1. Further­more, since the integral for large |F| vanishes like F-1 we must choose

m + n > —1

in order for q to vanish at infinity. It follows from (5-66) that in the neighborhood of the leading edge

q ~ Y~m~112, (5-68)

whereas near the trailing edge

q ~ (c – Y)-n~112. (5-69)

From the latter it follows that the Kutta-Joukowsky condition of finite velocity at the trailing edge is fulfilled only if n < —1. Hence from what was said earlier the only possible choice is

n = -1. (5-70)

From m we then find from (5-67) that it cannot be less than zero. It seems reasonable from a physical point of view that the lowest possible order of singularity of the leading edge should be chosen, namely

m = 0. (5-71)

However, from a strictly mathematical point of view there is nothing in the present formulation that requires this choice; thus any order singu­larity could be admissible. In settling this point the method of matched asymptotic expansions again comes to the rescue. The present formulation holds strictly for the outer flow only, which was matched to the inner flow near the airfoil. However, as was pointed out in Section 5-2, the simple inner solution (5-25) obviously cannot hold near the leading edge since there the ж-derivatives in the equation of motion will become of the same order as z-derivativ’es. To obtain the complete solution we therefore need to consider an additional inner region around the leading edge which is magnified in such a manner as to keep the leading edge radius finite in the limit of vanishing thickness. Such a procedure shows (Van Dyke, 1964) that the velocity perturbations due to the lifting flow vanish as Y~~1/2 far away from the leading edge. Hence (5-71) is verified and consequently

which is a solution of the integral equation (5-58).

As a simple illustration of the theory the case of an uncambered airfoil will be considered. Then for the lifting flow

w0 = —a

and for (5-73) we therefore need to evaluate the integral

j = J_ / dx і I X Д

7Г Jo X — Xi С — X1

This rather complicated integral may be handled most conveniently by use of the analytical techniques employed above. Using analytical con­tinuation, (5-75) is first generalized by considering instead the complex integral

whose real part reduces to (5-75) for г = 0+. Now we employ Cauchy’s integral formula (5-45) with

and the same path of integration as considered previously (see Fig. 5-6). Thus

1+C2+C3 Y

Along the large circle C1 we find by expanding the integrand in Ff1

The integral over С2 cancels as before, whereas the contribution along C3 becomes

Taking the real part of this for z = 0+ we obtain

/ = -1 (5-81)

and, consequently, by introducing (5-74) into (5-73),

u(x, 0+) = a = щ(х). (5-82)

Hence the lifting pressure distribution

ДCp = Cp(x, 0—) – Cp(x, 0+) = 4u0(x) = 4a (5-83)

has a square-root singularity at the leading edge and goes to zero at the trailing edge as the square root of the distance to the edge. The same behavior near the edges may be expected also for three-dimensional wings.

The total lift is easily obtained by integrating the lifting pressure over the chord. An alternative procedure is to use Kutta’s formula

L = р{7«,Г.

The total circulation Г around the airfoil can be obtained by use of (5-72). Thus

– -"-far-

The path of integration around the airfoil is arbitrary. Taking it to be a large circle approaching infinity we find that

For the flat plate this leads to the well-known result

In view of the linearity of camber and angle-of-attack effects, the lift – curve slope should be equal to 2ir for any thin profile. Most experiments show a somewhat smaller value (by up to about 10%). This discrepancy is usually attributed to the effect of finite boundary layer thickness near the trailing edge, which causes the rear stagnation point to move a small distance upstream on the upper airfoil surface from the trailing edge with an accompanying loss of circulation and lift. This effect is very sensitive to trailing-edge angle. For airfoils with a cusped trailing edge (= zero

trailing-edge angle), carefully controlled experiments give very nearly the full theoretical value of lift-curve slope.

According to thin-airfoil theory, the lifting pressure distribution is given by (5-83) for all uncambered airfoils. Figure 5-7 shows a comparison between this theoretical result and experiments for an NACA 0015 airfoil performed by Graham, Nitzberg, and Olson (1945). The lowest Mach number considered by them was M = 0.3, and the results have therefore been corrected to M = 0 using the Prandtl-Glauert rule (see Chapter 7). The agreement is good considering the fairly large thickness (15%), except near the trailing edge. The discrepancy there is mainly due to viscosity as discussed above. It is interesting to note that the theory is accurate very close to the leading edge despite its singular behavior at x = 0 discussed earlier. In reality, ДCp must, of course, be zero right at the lead­ing edge.

With the aid of the Prandtl-Glauert rule the theory is easily extended to the whole subsonic region (see Section 7-1). The first-order theory has

Fig. 5-7. Comparison of theoretical and experimental lifting pressure distribu­tions on a NACA 0015 airfoil at 6° angle of attack. [Based on experiments by Graham, Nitzberg, and Olson (1945).]

been extended by Van Dyke (1956) to second order. He found that it is then necessary to handle the edge singularities appearing in the first-order solution carefully, using separate inner solutions around the edges; other­wise an incorrect second-order solution would be obtained in the whole flow field.