Aeolian tone

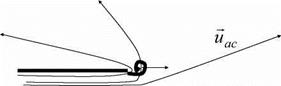
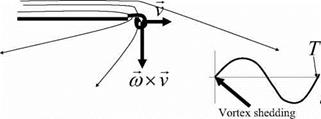
For Reynolds numbers ReD = UD/v above 50 the wake of a cylinder of diameter D placed with its axis normal to a uniform flow (velocity U, kinematic viscosity v) is unstable with periodic vortex shedding occurring. Vortices have alternating vorticity signs, which results in an oscillating lift force (normal to the flow direction). The force of this flow on the cylinder comes from a reaction force F of the cylinder on the flow. This reaction force acts as a source of sound. For low Mach numbers the cylinder is compact so that we can neglect variation of the retarded time over the source region. Equation (94) can be written in the following form:

xi dFj

4^cq|X|2 dt

The lift force scales with pU2DL where L is the length of the cylinder over which the vortex shedding is coherent. The oscillation frequency corre­sponds to a Strouhal number which is somewhat dependent on the Reynolds number SrD = fD/U « 0.2. This was already observed by Strouhal (1878) (Figure 15). The most important conclusions that can be drawn from this experience is:

• the cylinder does not need to vibrate or oscillate in order to generate the whistling tone,

• the vortices shed by the cylinder do not impinge on any surface or edge.

The first statement contradicts our intuition that sound is produced by wall vibrations. Oscillation of the cylinder can occur and can strongly affect the frequency. This occurs when the mechanical oscillation frequency is close to the natural vortex shedding frequency. One can then observe a strong increase in coherence length of the vortex shedding [Blake (1986)].

The second statement contradicts the intuition that sound is produced upon impingement of vortices on edges. In early work [Rockwell (1983)] this assumption is generally accepted, although it is a rather misleading assumption, as the following examples show.

5.5

Human whistling

As we have seen in section (6.4) convective effects induce acoustical en­ergy absorption upon reflection at an open pipe termination with outflow. The ratio of reflected and incoming acoustic intensity is, following equation (178) and with R = —1:

This is a consequence of the losses in total enthalpy AB’ = UU in the free jet formed by flow separation at the pipe exit. This corresponds to the modulation of the kinetic energy in the jet. This kinetic energy is dissipated by turbulence in the jet with negligible pressure recovery (p’ = 0).

We now analyse the same phenomenon by using the energy corollary of Howe (193). As a first step we consider the spatial distribution of the acoustic velocity field U at the pipe exit. A potential flow such as the acous­tic field u’ = Vp’ does not separate from sharp edges. This flow follows
the walls smoothly. The acoustic streamlines around the edges of the open pipe termination are curved. Which implies that there should be a pressure gradient directed towards the inner side of the bend which provides the centripetal force bending the streamlines. Following the Bernoulli equation (36) this decrease in pressure implies an increase of velocity towards the interior of the bend. Actually, this also follows directly from the condition that the acoustic flow should be irrotational Vx и’ = Vx V(f’ = 0. Rota­tion due to the bending of streamlines should be compensated by a gradient in the radial direction of the tangential component of the velocity. In terms of forces the radial pressure gradient balances the centrifugal force. As the velocity increases and the radius of curvature of the streamlines decreases as we approach the interior of the bend, the centrifugal force increases dra­matically. Obviously for a sharp edge we have a singularity in a potential flow [Prandtl (1934), Milne-Thomson (1952), Paterson (1983)]. As we ap­proach the edge, the magnitude of the acoustic velocity becomes infinitely large. However, moving away from the edge in the direction of the pipe axis, causes rapid decrease of the amplitude of the acoustic field. The direction of the acoustic velocity also turns gradually from normal to the pipe axis to­wards the direction of the pipe axis (Figure 16). A harmonically oscillating acoustic field implies that the acoustic flux is directed pipe-outward during half the oscillation period and is directed pipe-inward during the next half period.

The next step in our analysis is to consider the vortex shedding. Vortex shedding is the result of viscous effects in the boundary layers of the flow. In these boundary layers the flow velocity |v| decreases from the bulk flow velocity down to the zero velocity imposed by the no-slip boundary condition v = 0 at the wall. At high Reynolds numbers the boundary layers are thin. The flow in these boundary layers is mainly directed along the wall and this implies that the pressure in the boundary layer is equal to the pressure imposed by the bulk flow at the outer edge of the boundary layer. In the bulk of the flow there is an equilibrium between inertia and pressure gradient (as the viscous forces are negligible for high Reynolds numbers). An increase in pressure is compensated by a reduction of velocity. This allows fluid particles to move against an adverse pressure gradient. In the viscous boundary layer, the fluid has lost much of its kinetic energy and cannot use its inertia to overcome an adverse pressure gradient. Viscous drag of the fluid in the boundary layer by the bulk flow can allow to overcome a small pressure gradient. However, in a steady flow with bulk velocity U there will be back flow along the wall, when the characteristic time for momentum diffusion across the boundary layer ff2 /v (with ff the momentum thickness

Figure 16. Acoustic streamlines at an unflanged pipe termination.

of the boundary layer) becomes larger than the characteristic deformation time (dU/dx)-1. As a consequence the flow will separate from the wall. As the flow passes a sharp edge at the end of an unflanged open pipe, the flow will certainly separate from the wall. This implies that the flow continues tangentially to the wall (along the direction of the axis of the pipe) rather than following the bend, as does the potential flow. A shear layer is formed separating the main flow from a dead water region around the free jet. In this shear layer there is vorticity ш. Due to the instability of the shear layer this vorticity concentrates in coherent vortical structures (vortices). Each time the acoustic field turns from pipe inward to pipe outward a new vortex is formed at the edge of the pipe termination. This vortex accumulates most of the vorticty shed at the sharp edge while travelling at almost constant velocity Uc « U/2 in the direction of the pipe axis. The strength of the vortex is measured by the circulation Г = <fC v ■ dx = fS ш ■ dS taken along a contour C enclosing the vortex. The circulation is the flux of the vorticity vector through a surface sustained by the contour. In first approximation, with the acoustic velocity considerably smaller than the bulk flow velocity, we have dr/dt = – UUc, as illustrated in Figure 17 [Nelson et al. (1983)]. After an oscillation period a new vortex is shed from the pipe edges and the
old vortex continues to travel at almost constant speed. Further downstream these vortex rings are eventually dissipated by turbulence.

Figure 17. Vorticity and circulation of a shear layer.

Considering a new vortex shed at the edges of the pipe, we can see that the vector Uxv is directed normal to the pipe axis in the direction away from this axis. In first approximation the convection velocity is v « (Uc, 0, 0). At this point in time the acoustic velocity U is oriented in the same direction and locally very large due to the singularity of the acoustic flow at the edge. Hence the triple product —p0(U x v) ■ U is very large and negative. The formation of a new vortex by acoustic excitation of the shear layer implies sound absorption, which seems quite logical. The less trivial message from the theory of Howe, is that, after half a period, the same vortex will start to generate sound, because the sign of the acoustic velocity changes while those of the rotation ш and of the convection velocity v do not change. The power produced in the second half period is much lower than the initial sound absorption because the growth in the vortex circulation is not able to compensate for the decrease in acoustic velocity amplitude and its rotation in the direction of the pipe axis (Figure 18). We obtained a result similar to the predicted sound absorption found when using the quasi-steady model.

The major gain is the understanding that there is a possibility of net

Figure 18. Sound absorption as a result of a strong initial absorption.

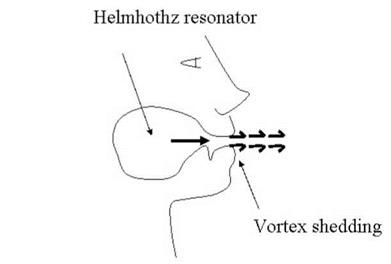
sound production by the vortex shedding, if we can reduce the initial ab­sorption and enhance production. This is exactly what occurs when we whistle with our lips. Flow separation at our lips occurs actually almost at the neck of the channel formed by our lips. This implies that there is a strong reduction of the singularity of the acoustic velocity, because the lips are rounded rather than sharp and the acoustic velocity is almost parallel to the axis of the flow. Moreover, if we ensure that the vortex travels over the radius of curvature R of our lips within half an oscillation period, it will start producing sound. As the acoustic field has not expanded in free space its amplitude is still large and the direction reasonably normal to the main flow axis. This particularly favourable condition is met when the Strouhal number SrR = fR/U = (fR/Uc)(Uc/U) « 0.25. The frequency f is im­posed by the Helmholtz resonance of our mouth cavity in combination with the neck formed by our lips (Figure 19) [Wilson et al. (1971), Hirschberg et al. (1995)]. Once we have adjusted this geometry we should tune the flow velocity to match the Strouhal number condition. This explains why a child, that is blowing too hard will never be able to whistle by blowing harder and harder.

This simple experiment confirms that we do not need any impingement of vortices on an edge to generate sound. It furthermore indicates that sharp

Figure 19. Human whistling [Wilson et al. (1971)].

edges are not necessary for vortices to be shed. Finally, it indicates that sharp edges at the flow separation point actually tend to reduce sound due to vortex shedding. We should note, however, that sharp edges will strongly enhance broadband noise production. This effect is clear when we consider the sound produced by blowing hard trough our lips in comparison with the sound produced by blowing along our teeth (as we do when we generate a fricative sound such as an [s]).

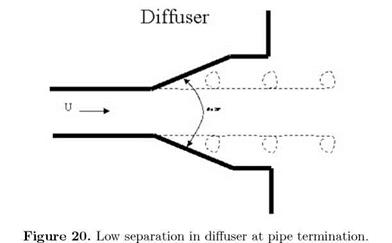
A related configuration is that of a pipe terminated by a diffuser, which is a conical expansion from the pipe cross section Sp to the outlet cross section S0. This allows reducing the loss of energy by dissipation of kinetic energy in the free jet at the outlet. This works only typically if the increase in cross section is not much larger than a factor 2. Furthermore, the opening angle of the diffuser cone should be less than 8 degrees. This would imply very long diffusers. In practice one uses therefore opening angles of about 20 to 25 degrees. In this case the flow partially separates from the wall within the diffuser. Considering the steady flow performance the losses due to this flow separation is rather marginal. However, it has a spectacular consequence on the energy reflection coefficient for acoustic waves travelling in the pipe towards the open end. Measurements of RE = I-/I+ appear to be larger than unity for two ranges of Strouhal numbers (Figure 20). This implies sound generation and potentially whistling of the pipe system too. The lowest Strouhal number corresponds roughly to a travel time of the vortices through the diffuser half that of the oscillation period. The second higher Strouhal number corresponds to a travel time of one and a half periods. This means that there are two co-existing vortices within the diffuser. These two flow conditions are called hydrodynamic modes or stages Howe (1998). The same type of behaviour can be observed with another configuration in the next section.