Jet Screech Tones

Experimental measurements have shown that screech tones are very sen­sitive to upstream conditions, as discussed in Section 2.3. The amplitude of the tone could be altered by as much as 10 dB, just by changing the nozzle lip thickness. It is not surprising then, that the prediction of screech amplitude is very difficult and no method for its prediction existed until recently. Shen and Tam (1998), Shen and Tam (2000), Shen and Tam (2002) have provided the first direct numerical simulations of axisymmetric and three-dimensional screech tones. The simulations use a finite difference

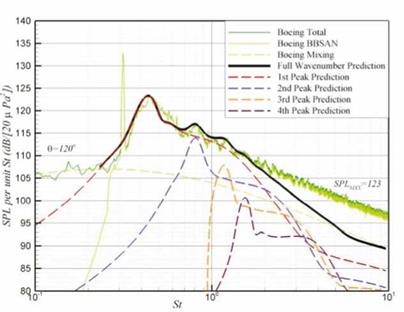
Figure 17. Total BBSAN prediction and the accompanying contributions from selective integrations over contributing wavenumbers of the shock cell structure wavenumber spectrum. Md = 1.0, Mj = 1.5, Tt/Ta = 1.0, R/D = 100, inlet angle = 60°. (From Morris and Miller (2010)).

methodology with optimized algorithms used for both space and time dis­cretizations. The effects of the fine-scale turbulence are included through the use of a к – є model. Comparisons of screech frequency, mode staging, and amplitude are made with experiments. Good agreement is obtained for all these phenomena. However, the calculations are computationally very expensive, even though three-dimensional effects are included in a simplified fashion. Thus, much work remains to be done in this area. On the other hand, several formulas for the prediction of the screech frequency have been developed over the years, based on different theoretical models.

4.1 Prediction of Screech Tone Frequency

The screech tone generation mechanism is very similar to the mechanism of broadband shock noise generation. For the generation of tones, a single excited instability wave is responsible, while for the generation of broadband

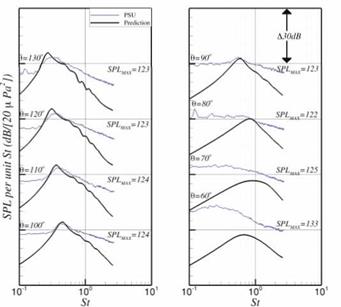
Figure 18. Comparison of BBSAN predictions with experiments for an AR = 1.75 rectangular jet. Md = 1.50, Mj = 1.70, Tt/Ta = 2.20, and R/De = 100, in the minor axis direction. (From Morris and Miller (2010)).

shock noise, a spectrum of instability waves is involved. Tam et al. (1986) examined the relationship between the two shock noise components and provided experimental and theoretical evidence that the two are indeed related. From the shock noise data of Norum and Seiner (1982a), they observed that the fundamental screech frequency was always at a lower value than the peak frequency of broadband shock noise and that the half-width of the broadband shock noise spectrum decreased rapidly as the observer position moved towards the jet inlet. They also showed that only a narrow band of frequencies are radiated in the upstream direction when acoustic waves are generated by the interaction mechanism. Based on their analysis and experimental observations, they suggested that the screech frequency could be regarded as the limiting case of broadband shock noise as the observer angle approaches the nozzle inlet, ф = 0°. The decrease in the spectrum half-width and peak frequency, and the approach of the broadband shock noise spectrum peak frequency to the screech frequency, is evident in

Figure 10.

Tam et al. (1986) also noted that the feedback mechanism was not similar to that for the generation of cavity tones or impingement tones since there was no constraining geometric feature that would set the feedback path length for a shock containing jet and thus set the frequency of the tone. They proposed that the screech frequency is determined by the weakest link of the feedback loop, which is the connection between the outer and inner parts of the loop at the nozzle exit. This connection is responsible for triggering the instability waves. Therefore, sound waves of sufficient strength must reach the nozzle exit in order to excite an instability wave of large enough amplitude, so as to maintain the feedback loop. However, the interaction mechanism generates only a narrow band of frequencies with high intensity that travel in the upstream direction. Hence if the feedback loop is to be self-sustaining, then the fundamental screech frequency must be confined to this upstream propagating narrow band of frequencies. The weakest link hypothesis also explains why a good approximation for the screech frequency is obtained by setting ф = 0 in the equation for the broadband shock noise peak frequency; Eqn. (62).

In order to use the expression for the peak frequency of broadband shock noise and screech, the value of the convection velocity uc and the shock cell wave number kn are required. As in the interaction theory, the source of broadband shock noise would occur near the axial location at which the instability wave attains its maximum amplitude, consistent with the obser­vations of Seiner and Yu (1984). Thus, the phase velocity and shock cell wavenumber must be evaluated at the location where the amplitude of the instability wave is maximum. This axial location can be determined if a locally parallel assumption for the mean flow is used in a stability analy­sis. Tam et al. (1986) describe an iterative procedure for the calculation of the fundamental screech frequency. This methodology requires no empiri­cal inputs and the screech frequencies are calculated from first principles. However, this methodology involves extensive computations. In order to develop a simple formula for the estimation of screech frequencies, they adopted some simplifying assumptions. First, on the basis of experimental observations, the convection velocity was assumed to be 70% of the fully expanded jet velocity Uj. Secondly, the value of the lowest order shock cell wave number, ki was obtained using a vortex sheet model for the shear layer. Additionally, to account for the finite thickness of the jet shear layer, the shock cell spacing near the end of the potential core was estimated to be 20% less than that given by the vortex sheet model. Using values based on these assumptions and the isentropic relationships, Tam et al. (1986) de­veloped the following semi-empirical expression for the fundamental screech

frequency,

In this expression, Dj is the fully-expanded jet diameter, which, as shown by Tam and Tanna (1982), is related to the nozzle geometric diameter D by,

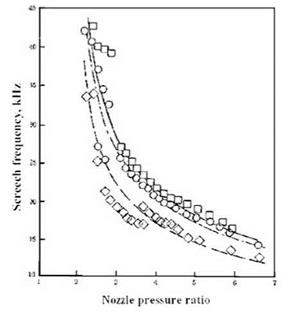
This formula was shown to provide good agreement with measured screech frequencies. Figure 19 from Tam et al. (1986) illustrates that Eqn. 64 pro­vides a good match with data obtained by Rosfjord and Toms (1975) using a convergent nozzle for both cold and heated jets. The agreement is better at higher nozzle pressure ratios. In the development of Eqn. (64), a helical instability wave mode was assumed to be dominant. It is known that for for a convergent nozzle and Mj < 1.3 the toroidal instability mode is dominant. Thus, it is perhaps not surprising that the agreement is not as good at the lower Mach numbers. Tam (1988) extended these concepts to jets of non – axisymmetric cross-sections and developed a formula for the calculation of screech tones from rectangular jets. Again, he demonstrated good agree­ment with measured frequencies. Morris et al. (1989) also used their shock cell model for arbitrary geometry jets to predict the screech frequencies for rectangular jets and obtained good agreement with measurements. Tam (1995a) developed an expression that accounted for the effect of forward flight on the peak frequency of radiation.

Recently, Panda (1998), Panda (1999) carried out detailed experiments on the screech generation mechanism of choked axisymmetric jets. He found a partial interference of the downstream-propagating instability waves and the upstream-propagating acoustic waves along the jet boundary, result­ing in a standing wave pattern. A corresponding length scale, identical to that of the standing wavelength, was also observed in the jet shear layer. The new length scale was found to be approximately 80% of the shock cell spacing. This new length scale correlated the measured screech frequencies well. Interestingly, Tam et al. (1986) selected the same modifications to the shock cell length based on entirely different reasoning (the effects of growth of the jet shear layer on the shock spacing) in the development of the semi-empirical formula, Eqn. (64). However, it should be noted that the complete analysis by Tam et al. (1986), in which the shock cell spacing is determined by a multiple scales analysis, does not involve this assumption.

Figure 19. Predictions of screech frequency for hot and cold jets from a round convergent nozzle at different nozzle pressure ratios. Measurements shown by symbols and predictions shown by lines. b,—————– , Tt = 291 K; O,———— , Tt = 596 K; □,—————- , Tt = 803 K. (From Tam et al. (1986), with permission)