Equilibrium of the Glider (3-D Incompressible Flow)

15.1.3.1 Glider Effective Aspect Ratio

As a glider (engine off), the glider has the following lift and moment coefficients in terms of the geometric angle of attack a (rd) and tail setting angle tt (rd):

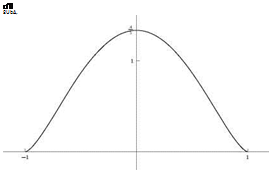Fig. 15.2 Distribution of circulation

Cl (a, tt) — 5.3a + 1.56 + 0.5tt
Cm, o (a, tt) — —1.7a — 0.43 — 0.45tt

The effective aspect ratio AR of the glider (wing+tail), assuming ideal loading is given by the lift slope

— 5.3

15.1.3.2 Equilibrium Angle of Attack

The equilibrium of the moment corresponds to

xcg

CM, o(aeq, tt) + 7—- CL (aeq, tt) — 0

lref

When the lift and moment coefficients functions are replaced in the above equa­tion, taking into account ^ — 0.29, the solution for aeq(tt) reads

Plugging this result in the lift coefficient gives

Cl (tt) — 5.3(0.137 — 1.87tt) + 1.56 + 0.5tt — 2.286 — 9.411tt

which for CL — 1.9 results in tt — 0.041 rd — 2.35°.

The corresponding angle of attack is aeq — 0.06 rd — 3.46°.

15.1.3.3 Landing Speed

At sea level, p — 1.2kg/m3, g — 9.81 ms—2, given that the total mass m — 15kg, the maximum lift coefficient (CL)max — 1.9 corresponding to the reference area Aref — 0.68 m2, the landing speed corresponds to the equilibrium equation for the force in the direction normal to the trajectory. i. e.

15.2 Solution to Problem 2