Compressible 2D far-field

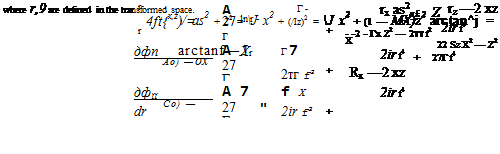Since the transformed flow problem is incompressible, we can re-use the far-field expressions for the far – field potentials and velocities which were developed in Chapter 2. For the 2D case we have

The four far-field coefficients can be defined directly from the incompressible definitions, with the reverse transformation immediately included to put them in terms of the physical parameters.

л = V*r* = V*S* e (8.110)

f = Iv^cct = ilLccif (8.111)

= lU(l + ^) = 1+^) (8.112)

Rz = cmo = ^KoC2cmo/l2 (8.113)

A complication in relating Л to the drag coefficient is that at high speeds the boundary layer and wake fluid is heated significantly via friction, which reduces its density relative to the potential flow. The reduced density increases S* relative to Г, as can be seen from comparing their definitions (4.4) and (4.11) for p/pe < 1. An approximate relation between the far-downstream thicknesses is

S* ^ (l + (Y—1 )M*) Г* (8.114)

which follows from the assumption that the wake has a constant total enthalpy, as discussed in Section 1.6. The far-field source (8.110) can then be more conveniently given in terms of the profile drag coefficient cd = 2Г*/с as follows.

A = hA /3 (1 + (7-l)M2 ) = ^ccd /З (1 + (7-l)M2 ) (8.115)

With all the transformed far-field coefficients known, the transformed perturbation potential and velocities can be calculated from (8.105),(8.106),(8.107) at any field point of interest. The physical perturbation potential and velocities are then obtained by the usual reverse transformations:

One complication with this treatment is the far-field contribution of the higher-order compressibility terms in the PP2 equation (8.55), which are ignored in the first-order PG equation. Specifically, the source and vortex parts of (ff in (8.105) have their own field-source distributions as given by (8.1), which then should be included in the fx integral (8.104) above, and the corresponding fz integral as well. This correction is treated by Cole and Cook [59]. The main effect is that an airfoil’s far-field x-doublet now also depends to some extent on its lift, not just on its area.