LONGITUDINAL CONTROL

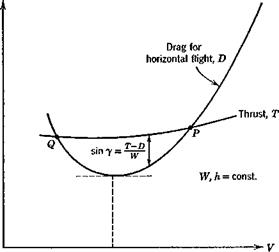
The two principal quantities that need to be controlled in symmetric flight are the speed and the flight-path angle, that is to say, the vehicle’s velocity vector. To achieve this obviously entails the ability to apply control forces both parallel and perpendicular to the flight path. The former is provided by thrust or drag control, and the latter by lift control via elevator deflection or wing flaps. It is evident from simple physical reasoning (or from the equations of motion) that the main initial response to opening the throttle (increasing the thrust) is a forward acceleration, i. e. control of speed. The main initial response to elevator deflection is a rotation in pitch, with subsequent change in angle of attack and lift, and hence the development of y, a rate of change of flight-path angle. When the transients that follow such control actions have ultimately died away, the new steady state that results can be found in the conventional way used in performance analysis. Fig. 10.1 shows the basic relations. The steady speed V at which the airplane flies is governed by the lift coefficient, which is in turn fixed by the elevator angle—see (6.4,13). Hence a constant 6e implies a fixed V. The flight-path angle у at any given speed is determined, as shown in Fig. 10.1, by the thrust. Thus the ultimate result of moving the throttle at fixed elevator angle (when the thrust line passes through the C. G.) is a change in у without change in speed. But we saw above that the initial response to throttle is a change in speed—hence the short-term and long-term effects of this control are quite contrary. Likewise we saw that the main initial effect of moving the elevator is to rotate the vehicle and influence y, whereas the ultimate effect at fixed throttle is to change both speed and y. The short-term and long-term effects of elevator motion are therefore also quite different. The total picture of longitudinal control is clearly far from simple, and the transients that connect the initial and final responses require investigation. We shall see in the

following that these are dominated by the long-period, lightly damped phugoid oscillation, and that the final steady state with step inputs is reached only after a long time. These matters are explored more fully in the following sections.