A COMPLEX ^ UPPER BOUND
![]() Introduction of D scales in the standard interconnection structure
Introduction of D scales in the standard interconnection structure
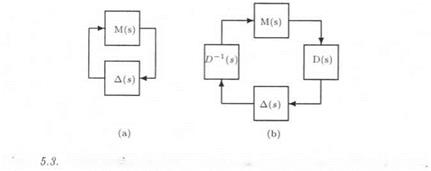
The standard interconnection structure M(s) — A(s) of Figure 5.3.a is considered. A p upper bound at frequency u> is directly provided by the small gain theorem (M = M(juj)):
p(M) < o(M) (5.8)
 This theorem does not account for the structure of the model perturbation A(s). To this aim, a scaling transfer matrix l)(s) is used to decrease
This theorem does not account for the structure of the model perturbation A(s). To this aim, a scaling transfer matrix l)(s) is used to decrease
the conservatism of the /л upper bound (5.8) (Safonov, 1982). D(s) must commute with A(s), i. e.:
D~1{s)A{s)D{s) = A{s) (5.9)
D(s) can thus be introduced into the interconnection structure without modifying the stability properties of the closed loop (see Figure 5.3.b).
Frequency и is fixed: let then D = D(ju) and M = M(jiv). Remember that the structure of the model perturbation A is (see equation (1.26)):
where J[ and Jj are real and complex scalars, while Д^ Є Ckrnr+mc+4,kmr+m<:+’> is a full complex block. Because of equation (5.9), the scaling matrix D must belong to:
![]() , Dmr+mc i + i ) ■ * • > dmc ^mr-fmc+mc )
, Dmr+mc i + i ) ■ * • > dmc ^mr-fmc+mc )
with Di = D* >0,Die Cki’ki and dj > 0} (5.11)
The new fj. upper bound is:
![]() ц(М) < mf^a(DMD~l)
ц(М) < mf^a(DMD~l)
The issue is thus to minimize the conservatism of this upper bound by finding the optimal value of D, which minimizes a{DMD~l). This convex and non differentiable optimization problem can be solved in numerous ways: see (Doyle, 1982; Safonov, 1982; Packard and Doyle, 1993) and included references.
Remark: this jr upper bound can be rewritten as1:
This is an LMI problem involving the minimization of a maximal generalized eigenvalue.











