The Piston Theory

The piston theory is an approximate theory which works for thin wings at high speeds and at small angles of attack. If the characteristic thickness ratio of a body is s and Ms is the hypersonic similarity parameter then for Ms ^1 the Newtonian impact theory works well. For the values of Ms < 1 the piston theory becomes applicable. Since s is small for thin bodies, at high Mach numbers the shock generated at the leading edge is a highly inclined weak shock. This makes the flow region between the surface and the inclined shock a thin boundary layer attached to the surface. If the surface pressure at the boundary layer is p and the vertical velocity on the surface is wa, then the flow can be modeled as the wedge flow as shown in Fig. 1.9.

The piston theory is based on an analogy with a piston moving at a velocity w in a tube to create compression wave. The ratio of compression pressure created in the tube to the pressure before passing of the piston reads as (Liepmann and Roshko 1963; Hayes and Probstien 1966)

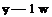
_P_

pi

Here, ax is the speed of sound for the gas at rest. If we linearize Eq. 1.30 by expanding into the series and retain the first two terms, the pressure ratio reads as

— ffi 1 + у— (1.31)

pi ai

Wherein, wa is the time dependent vertical velocity which satisfies the following condition: wa ^ аж. The expression for the vertical velocity in terms of the body motion and the free stream velocity is given by

Equation 1.31 is valid only for the hypersonic similarity values in, 0 < Ms < 0.15, and as long as the body remains at small angles of attack during the motion while the vertical velocity changes according to Eq. 1.32. For higher values of the

hypersonic similarity parameter, the higher order approximations will be provided in the relevant chapter.