Initial and Boundary Conditions

The study of aerodynamical problems with real gas effects requires solution of a system of partial differential equations which are first order in time and second order in space coordinates. In order to solve Eq. 2.49 to determine the flow field, all dependent variables must be prescribed at time t = 0, and for all times t at the boundaries of the computational domain. All the prescribed values must be in accordance with the physics of the problem. As the initial conditions for the unknown values of U we prescribe the undisturbed flow conditions, i. e., u = 1, v = w=0 which represents the impulsive start of the flow. Under these conditions the initial values for the unknown vector in generalized coordinates become fp0

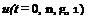
p0

0

0

£°

c0J

Here, po is the initial value for the density, eo is the initial value for the energy and cf is the initial value of the ith specie.

As for the boundary conditions: (i) the unknowns at the surface, and (ii) farfield boundary conditions must be provided.

Accordingly:

i. As the no slip condition at the surface: U(t, i, g, 1 = 0) = 0 is prescribed. (In Fig. 2.6, 1 = 0 prescribes the surface). In reactive flows the catalicity of the surface determines the value of the concentration gradients,

ii. At the farfield: for 1 = imaks U(t, П, g, 1 = 1maks) = U 1 is prescribed, and the flux condition at П = imaks is Щ = (0),

iii. If there is a symmetry condition as shown in Fig. 2.6b, we prescribe the flux normal to the symmetry as Щ = (0).