Using Orthogonality

The property of orthogonality is useful in many aspects of structural-dynamics anal­ysis. As an illustration, consider the response of an unforced uniform string to initial conditions. In this case, there are no external loads on the string, but it is presumed to have an initial deflection shape and an initial velocity distribution. Let these initial conditions be represented as

v(x, 0) = f(x) dv

dfi (x’ 0) = g(x)

where both f (x) and g(x) must be compatible with the boundary conditions.

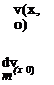Using Eq. (3.21), these initial conditions can be written in terms of the modal representation as

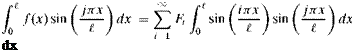Both of these relationships are multiplied by sin (jnx/l) dx and integrated over the length of the string. The first relationship yields

(3.46)

Fjl

2

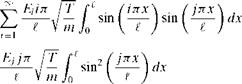where the evaluation used the orthogonality property of the mode shapes, which causes every term in the infinite sum to be zero except where i = j. The mass per unit length is constant for this case and hence does not appear under the integral. The second relationship can be reduced in a similar manner, so that

Ejj n T

2 V m

This treatment of the initial conditions therefore permits a direct evaluation of the unknown constants (Ei and Fi) in the modal representation of the total string displacement; that is

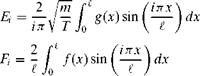(3.48)

Thus, for the prescribed initial conditions given by f (x) and g(x), the resulting string displacement can be described as

TO

v(x, t) = ^2 sin

i =1

Example: Response Due to Given Initial Shape. To further illustrate this proce­dure, consider the case of the plucked string with zero initial velocity. Let the initial shape be as shown in Fig. 3.2. If we assume the initial velocity to be zero, then g(x) = 0 and Ei = 0 for all i. The string displacement becomes

v(x, t) = F. sin l-^ cos(«ii) (3.50)

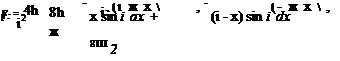Substitution of this function into the preceding integral yields

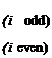It may be noted that sin(i n/2) is zero for all even values of the index and that it is either +1 or -1 for odd values. If desired, these constants can be written as

The fact that F. = 0 for all even values of i is indicative of the symmetry of the initial string displacement about the midpoint. That is, because the initial shape is symmetric about x = i/2, no antisymmetric modes of vibration are thereby excited. The total string displacement becomes

8h (-1)L-r, (iжx

v(x, t) = —2 T2—sin i cos(«;t) (3.54)

n i=1,3,… i Vі/

where

i ж T і m

It should be noted from this solution that the modal contributions to the total displacement significantly decrease as the mode number (i. e., the index i) increases. This can be observed by the dependence of Fi on i and is characteristic of almost all structural-dynamics response problems; thus, it permits a truncation of the infinite sum to a finite number of the lower-order modes. This solution indicates that the string will vibrate forever, with the string periodically returning to its initial shape. In actual systems, however, there are always dissipative phenomena that cause the motion to die out in time. This is considered when we address aeroelastic flutter in Chapter 5.