Wings of finite span

When the component of the free-stream velocity perpendicular to the leading edge is greater than the local speed of sound the wing is said to have a supersonic leading edge. In this case, as illustrated in Fig. 6.56, there is two-dimensional supersonic flow over much of the wing. This flow can be calculated using supersonic aerofoil theory. For the rectangular wing shown in Fig. 6.56 the presence of a wing-tip can only be communicated within the Mach cone apex which is located at the wing-tip. The same consideration would apply to any inboard three-dimensional effects, such as the ‘kink’ at the centre-line of a swept-back wing.

The opposite case is when the component of free-stream velocity perpendicular to the leading edge is less than the local speed of sound and the term subsonic leading edge is used. A typical example is the swept-back wing shown in Fig. 6.57. In this case the Mach cone generated by the leading edge of the centre section subtends the whole wing. This implies that the leading edge of the outboard portions of the wing influences the oncoming flow just as for subsonic flow. Wings having finite thickness and incidence actually generate a shock cone, rather than a Mach cone, as shown in

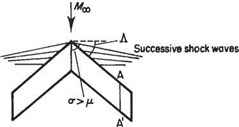Fig. 6.57 A wing with a subsonic leading edge

Fig. 6.58

Fig. 6.58. Additional shocks are generated by other points on the leading edge and the associated shock angles will tend to increase because each successive shock wave leads to a reduction in the Mach number. These shock waves progressively decelerate the flow, so that at some section, such as AA’, the flow approaching the leading edge will be subsonic. Thus subsonic wing sections would be used over most of the wing.

Wings with subsonic leading edges have lower wave drag than those with super­sonic ones. Consequently highly swept wings, e. g. slender deltas, are the preferred configuration at supersonic speeds. On the other hand swept wings with supersonic leading edges tend to have a greater wave drag than straight wings.