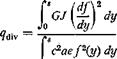SOLUTION BY GENERALIZED COORDINATES

An alternative procedure based on the semirigid assumption is to apply the concept of generalized coordinates. This point of view provides a new ground for refinements in the calculation.

Let us again consider the idealized problem of § 3.2. Assume that the angle of twist along the span is a known function of у with unspecified amplitude. Let us write

%) = 0of(y) (1)

* For a uniform beam, the angle of twist due to a concentrated torque applied at the tip (reference) section varies linearly along the span. So the assumption (Eq. 11) is consistent with the influence coefficients, and with the stiffness constant К given by Eq. 9.

where 60 is an undetermined constant and f(y) is a given function. We shall consider в0 as the generalized displacement of the wing. The strain energy due to torsion is
(2)
The equation of equilibrium may be obtained from Lagrange’s equation
£ dt	dV л + w~Q
(3)
where the generalized force Q is to be found as follows: The aerodynamic twisting moment about the elastic axis, per unit span, is, according to the strip assumption, Ma’ = qc2ae0 = qc2ae60f(y) By permitting a variation of the amplitude 660, the corresponding virtual work is Q <50o = f Ma'(y) 6 %) dy = f qc2ae60f(y) 660f(y) dy Jo Jo Hence, Q == ?0O fi2ae f%y) dy (4)
At the critical-divergence speed, the wing is neutrally stable. A disturbed configuration can be maintained without causing any motion. Hence the kinetic energy T vanishes, and Eq. 3 implies
f C2aef2(y) dy Jo
(5)
Therefore,
Jo
(6)
Let GJ, c, a, e be constants, and assume f(y) — y/s. Then
Example 1.
s 3

Hence,

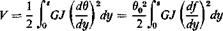
3 GJ c^aes1

which agrees with the result in § 3.3.

Example 2. Let GJ, c, a, e be constants, and assume

,, , . -ny

|o/4s)rfs-{*in"f*/ = 5

AV) = sm —

Hence,

n4JJ

which agrees with the exact result. In the present approach, a correct assumption of the semirigid mode yields an exact solution.

The procedure can be generalized as follows: Let

П

%)в і Ш) о)

t = о

wheref({y) (/ = 0, 1, 2, • • •, n) are known functions, and are generalized coordinates. Then

Qi = ( Ma'(y)f№ dy И = 0, 1,2, • • ■, n)

Jo

At the critical-divergence speed,

(/ = 0, 1, 2, • • •, n) (9)

Equation 9 gives a set of homogeneous linear simultaneous equations in Since the вt are, by assumption, not all zero, the determinant of the coefficients of these equations must vanish. This determinantal equation

involves the dynamic pressure qd{v. Its real positive roots are the critical values.

In calculating the aerodynamic moment Ma’ in Eq. 8, a theory may be selected, such as Prandtl’s lifting-line theory or Weissinger’s theory. If we use the strip assumption, then

П

М: = qdh, c2ae^J), fiiy) (10)

1 = 0

Finally, let us remark that, if the functions f{(y) (i = 0, 1, 2, • • •) are infinite in number and form a complete set,* then in the limit n -> oo an exact solution can be obtained.!

Example. Let GJ, e, c, a be constants across the span, and assume

%) = Яі – + Я2~-2

S. Vs

Then

+ 26A + 022)

By strip theory,		M; = q*ae (^ + 7**1)
Hence,
	0i + —2 02) “ ty — qc^aes f (J70x + drl s / s Jo

= ^aes (I + f)

Similarly,

Q2 = qc2aes

* A set of functions fjy), a < у < b, (i = 0, 1, 2, • • •) is said to be “complete” if an arbitrary function u{y) can be expanded into a generalized “Fourier” series of the

OO

formic,/to). i=o

t If the function fly) in Eq. 1 is regarded as unknown, the equation governing/(2/) can be derived from Eq. 5 by considering the first variation of that equation with respect to f(y). The result is the same as that obtained in § 3.2.

Let

1 = GJ^aec2si

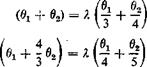Then the equations of equilibrium 9 become

A nontrivial solution exists only if the determinantal equation

is satisfied. The two roots are X = 2.48 and 32.3, corresponding to

2.48 G/ 32.3GJ

—– гт and ———-

The first one is the “fundamental” divergence dynamic pressure. Com­pared with the exact value given by Eq. 17 of § 3.2, the error is 0.6 per cent too large.