The Radiation-Adiabatic Vehicle Surface

3.2.1 Introduction: Surface Radiation Cooling

Surface (thermal[17]) radiation cooling of the external vehicle surfaces is the basic cooling mode of high-speed vehicles, either RV’s or CAV’s/ARV’s, op­erating in the Earth atmosphere at speeds below 8 km/s. At these speeds radiation emission and absorption processes of heat in the air-stream past the vehicle can be neglected. Nevertheless, we have a closer look at radiation emission from a bow-shock layer.

Radiative heating by heat emission from the gas in the bow-shock layer due to the high compression and the resulting rise of the gas temperature in that region potentially is the most important source of radiation towards the surface.4 The effect is strongest in the stagnation point region of a body, which can have a rather large extension, if a RV at high angle of attack is considered.

In order to show that shock-layer radiation indeed can be neglected in the flight situations considered here, we compare the ordinary stagnation-point (s) heat flux qgw, s with the heat flux due to shock-layer radiation qrad, s. We employ simple engineering formulas.

11.03- 10[18]

For the stagnation-point heat flux of a sphere with radius R a good ap­proximation to the relation of J. A. Fay and F. R. Riddell, [9], is given by R. W. Detra and H. Hidalgo, [10], (see also [11]):

With the nose radius R [m], the speed v[m/s], and the circular orbit velocity vco = 7.95-103 m/s, we get qgw, s [W/cm2].

7.9 • 107 R

^12-5 10[19]У

For the estimation of the radiative heat flux from the shock layer to the body surface we use a formula from [12] for flight speeds v^ 6,000 m/s with the same dimensions as in eq. (3.9)

It is important to note that this shock-layer radiation heat flux, qrad, s, to the blunt-nose surface is directly proportional to the nose radius R, whereas the heat flux in the gas at the wall, qgWyS, is proportional to 1//Д.

We compare now the two heat fluxes for a speed of v= 8-103 m/s at 90 km altitude, where we have p= 3.416 – 10~6 kg/m3. With a nose radius of the body R = 1 m and the density at sea level pSL = 1.225 kg/m3 we obtain

This shows that indeed shock layer radiation towards the body surface can be neglected at speeds below « 8 km/s in the Earth atmosphere below « 100 km altitude.

If, for whatever reason, radiation cooling is not sufficient, other (addi­tional) cooling means, for instance transpiration cooling, ablative cooling etc., must be employed. Usually this results in extra weight, enlarged system complexity, or restricted re-usability of the vehicle.

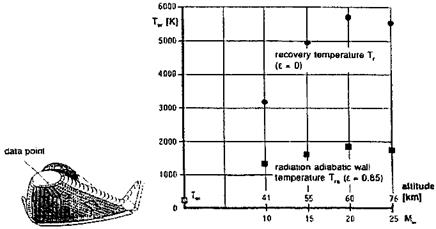
Surface radiation cooling is very effective. Fig. 3.2 shows with data com­puted by F. Monnoyer that radiation cooling reduces the wall temperature of a re-entry vehicle by such a degree that present-day TPS materials can cope with it without additional cooling [13, 14]. Without radiation cooling (emissivity є = 0), the recovery temperature Tr near the stagnation point of the HERMES re-entry vehicle reaches around 6,000 K at approximately 70 km altitude.[20] With radiation cooling (emissivity є = 0.85), the wall temper­ature Tw = Tra near the stagnation point is nearly 4,000 K lower than Tr, and remains below 2,000 K on the entire trajectory.

Fig. 3.2. Effect of radiation cooling at a location close to the stagnation point of HERMES (x = 1 m on the lower symmetry line, a = const. = 40°), laminar flow, equilibrium real-gas model, different trajectory points (MTO, altitude H) [13] (coupled Euler/second order boundary-layer method [14]).