Climbing

During level flight the power of the engine must produce, via the propeller, jet or rocket, a thrust equal to the drag of the aeroplane at that particular speed of flight. If now the engine has some reserve of power in hand, and if the throttle is further opened, either –

(a) The pilot can put the nose down slightly, and maintain level flight at an increased speed and decreased angle of attack, or

(b) The aeroplane will commence to climb (Fig. 7B).

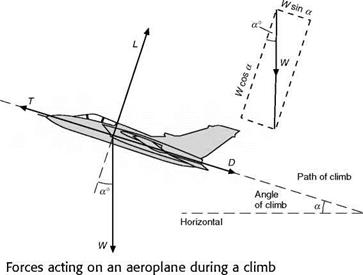
A consideration of the forces which act upon an aeroplane during a climb is interesting, but slightly more complicated than the other cases which we have considered.

Fig 7B Climbing

An Airbus A-330 using flaps to demonstrate its steep climb capability.

Assuming that the path actually travelled by the aeroplane is in the same direction as the thrust, then the forces will be as shown in Fig. 7.1. If a is the angle of climb, and if we resolve the forces parallel and at right angles to the direction of flight, we obtain two equations –

(1) T = D + W sin a (2) L = W cos a

Translated into non-mathematical language, the first of these equations tells us that during a climb the thrust needed is greater than the drag and increases with the steepness of the climb. This is what we would expect. If a vertical climb were possible, a would be 90° and therefore sin a would be 1, so the first equation would become T = D + W, which is obviously true because in such an extreme case the thrust would have the opposition of both the weight and the drag. Similarly if a = 0° (i. e. if there is no climb), sin a = 0. Therefore W sin a = 0. Therefore T = D, the condition which we have already established for straight and level flight.