Conservation Laws of Aerodynamics

In the general approach to the problem, it will be assumed that the flow through the rotor is one-dimensional, quasi-steady, incompressible and inviscid. Consider first an ideal fluid, that is, one that generates no viscous shear between fluid elements. Therefore, induced losses are the sole source of losses in the fluid, with other losses resulting from the action of viscosity being assumed negligible, at least for now. Furthermore, assume that the flow is quasi-steady, in that the flow properties at a point do not change with time. Finally, assume that the flow is one-dimensional, and so the properties across any plane parallel to the rotor plane are constant; that is, the fluid properties change only with axial (vertical) position relative to the rotor.

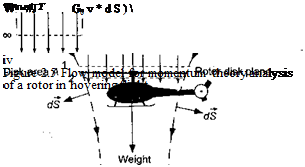
Consider the hover problem. Let the control volume surrounding the rotor and its wake have surface area S, as shown in Fig. 2.5. Let dS be the unit normal area vector (i. e., the outward facing normal), which by convention always points out of the control volume across the surface S. A general equation governing the conservation of fluid mass applied to this finite control volume can be written as

(2.1)

where V is the local velocity and p is the density of the fluid. This equation states that the mass flow into the control volume must equal the mass flow out of the control volume. Notice that this is a scalar equation. Similarly, an equation governing the conservation of fluid momentum can be written as

For an unconstrained flow, the net pressure force on the fluid inside the control volume is zero. This point has been considered by Glauert (1935), although it is not so obvious. Therefore, the net force on the fluid, F, is simply equal to the rate of change with time of the fluid momentum across the control surface, S. Although Eq. 2.2 is a vector equation, it can be simplified considerably by the assumptions of quasi-one-dimensional flow. This is essentially a byproduct of assuming a uniform pressure jump over the rotor disk and leads to uniform distributions of velocity across any horizontal cross section within the control volume. Because the force on the fluid is supplied by the rotor, by Newton’s third law the fluid must exert an equal and opposite force on the rotor. This force is the rotor thrust, T. Finally, an equation governing the conservation of energy in the flow can be written as

This equation states simply that the work done on the fluid by the rotor manifests as a gain in kinetic energy of the fluid in the rotor slipstream per unit time. It is also a scalar equation.