Comparison of Theory with Measured Rotor Performance

In terms of coefficients it is apparent that the ideal power according to the simple momentum theory can be written as

/ґ 0^74

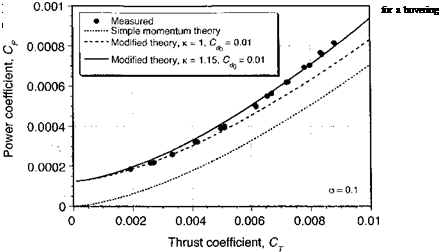
Figure 2.7 shows a comparison of the simple momentum theory with thrust and power measurements made for a hovering rotor using Eq. 2.37. Notice that the momentum theory underpredicts the actual power required, but the predicted trend that Cp ос Сът/2 is essentially correct. These differences between the momentum theory and experiment occur because viscous effects (i. e., nonideal effects) have been totally neglected so far.

2.4 Nonideal Effects on Rotor Performance

kC3t/2 V2 ’

In hovering flight the induced power predicted by the simple momentum theory can be approximately described by an empirical modification to the momentum result in Eq. 2.34, namely

where к is called an induced power correction factor or just an induced power factor. This coefficient is derived from rotor measurements (see Section 2.9) or flight tests and it encom­passes a number of nonideal, but physical effects, such as nonuniform inflow, tip losses, wake swirl, less than ideal wake contraction, finite number of blades, and so on. For prelim­inary design, most helicopter manufacturers use their own measurements and experience to estimate values of к, a typical average value being about 1.15. Values of к can also be computed directly using more advanced blade element methods (see Chapters 3 and 10), where the effects of the actual flight condition can be more accurately represented. This is

particularly important for high-speed forward flight, where the increasing nonuniformity of the inflow from reverse flow on the retreating blade must be accounted for.

Wake swirl effects serve to reduce the net change of the fluid momentum in the vertical direction and they will decrease the rotor thrust for a given shaft torque (power supplied) or will increase the rotor power required to produce a given thrust. Johnson (1980) shows that as a result of wake swirl the induced power is increased by a factor [1-f Ct ln(Cy /2) – b CV/2]-1; this is less than 1% at the values of Су typically found on helicopters and can be neglected as a contributor to rotor power requirements. However, see also the discussion of wake swirl in Section 3.3.6.

Proper estimates for the profile power consumed by the rotor requires a knowledge of the drag coefficients of the airfoils that make up the rotor blades; that is, a strip or blade element analysis is required. The airfoil drag coefficient will be a function of both Reynolds number, Re, and Mach number, M, which obviously vary along the span of the blade. A result for the profile power, Pq, can be obtained from an element-by-element analysis of sectional drag forces (i. e., the blade element method – see Chapter 3) and by radially integrating the sectional drag force along the length of the blade using

P0 = QNb f Dydy, (2.39)

Jo

where Nb is the number of blades and D is the drag force per unit span at a section on the blade at a distance у from the rotational axis. The drag force can be expressed convention­ally as

D = f>U2cCa = ір(ад2сС,, (2.40)

where c is the blade chord.. If the section profile drag coefficient, Q, is assumed to be constant (= Cdf) and independent oiRe and M (which is not an unrealistic first assumption), and the blade is not tapered in planform (i. e., a rectangular blade), then the profile power integrates out to be

P0 = – pNbtfcC^R4.

Converting to a standard power coefficient by dividing through by pA(QR)3 gives

(Nbcr _ 1

7TR)Cdo 8

The grouping NbcR/A (or Nbc/nR) is known as the rotor solidity, which is the ratio of blade area to rotor disk area and is represented by the symbol a. Typical values of a for a helicopter rotor range between 0.05 and 0.12, and much use of this solidity parameter is made throughout this book.

Armed with these estimates of the induced and profile power losses, it is possible to recalculate the rotor power requirements by using the modified momentum theory result that

r3/2 r

Cp = CPi + CPo = K-±- + (2.43)

These alternative results are also shown in Fig. 2.7, as denoted by the “modified theory,” which has been calculated by assuming Cd0 = 0.01. In the first case, it has been assumed that к = 1.0 (ideal induced losses), and in the second case, к = 1.15 (nonideal losses).

The value of cr for this particular rotor is 0.1. Notice the need to account for nonideal induced losses to give agreement with the measured data. The overall level of correlation thus obtained gives considerable confidence in the modified momentum theory approach for basic rotor performance studies, at least in hover.