BASIC AERODYNAMIC FORCES AND MOMENTS

It is apparent from Section 3.2 that if we specify certain geometric parameters of the aircraft like reference surface area of the wings S, the mean aerodynamic chord C, the wing-span b, and the dynamic pressure q (Appendix A), then we have the following straightforward aerodynamic force and moment equations:

Force = pressure x area

Fx = CxqS

Fy = CyqS (4.1)

Fz = CzqS

These equations are in terms of the component forces in x, y, and z directions. Moment = force x lever arms (respective ones):

L = CiqSb

M = CmqSc (4.2)

N = CnqSb

These expressions are in terms of the component moments: rolling moment L about the x-axis, pitching moments M about the y-axis, and the yawing moment N about the z-axis (see Appendix A for geometry of b and qc used as appropriate lever arms for defining the moments). Here, Cx, Cy, Cz, Cl, Cm, and Cn are the nondimensional body-axis force and moment coefficients, and are called aerodynamic coefficients. They are the proportionality constants (that actually vary with certain variables) in Equations 4.1 and 4.2. The dynamic pressure is q = 1/2pV2 in terms of air density p
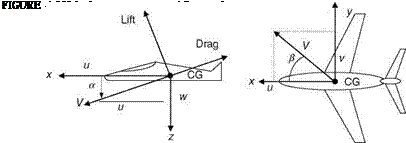
at an altitude and air speed V. Actually V is the total velocity of the air mass striking the vehicle at a certain (total) angle, and both the velocity V and the total angle can be resolved as shown in Figure 4.2 in terms of component velocities: u, v, and w and AOA and AOSS (Appendix A).

From the geometry of the flow velocity (Figure 4.2) the following expressions of the flow angles and component velocities emerge very naturally:

і w

a = tan

u

v

b = sin-1 V

as AOA (in vertical x—z plane) and AOSS (in horizontal x—y plane), respectively. By trigonometric transformation we get equivalent expressions as

u = V cos a cos b

v = V sin b (4.4)

w = V sin a cos b

The total velocity V of the airplane is expressed as

V = J u2 + v2 + w2 (4.5)

in terms of its components u, v, and w. From the basic definition of pressure and force, we have come to some more details of the interplay of flow angles, compon­ents of the total velocity, and aerodynamic coefficients. We must emphasize here that discussing the three components of the total velocity in the three directions is equivalent to considering that the airplane experiences motion along these three axes. Thus, we have put the airplane in real motion in air. What actually keeps the airplane lifted in the air is the lift force (Appendix A).

Now, if the values of the aerodynamic coefficients are known, then the forces and moments acting on the aircraft can be determined easily, since V and the geometrical parameters like area and lever arms are known. Alternatively, if we know the forces by some measurements on the aircraft, then the aerodynamic coefficients can be determined. Actually, the latter is true of the WT experiments conducted on a scaled model of the actual aircraft. In a WT, the model is mounted and compressed air is released, thereby putting the model in the flow field. The experiments are conducted at various AOA and AOSS settings, and forces and moments are measured/calculated from equivalent measurements. Since the flow velocity and the dynamic pressure are known, simple computations would lead to the determination of the aerodynamic coefficients. One can see that these coefficients can be obtained as a function of flow angles, Mach number, etc. Similar analysis can be done using CFD and other analytical methods (Appendix A). To determine the aerodynamic coefficients from experimental/flight data we need to know V and measure the force, i. e., force = mass x acceleration. Since mass is known, acceleration can be obtained as qSCx. Therefore, if the acceleration of an airplane in motion is measured, the aerodynamic coefficient Cx can be obtained. Since the coefficients depend on aerodynamic derivatives and dynamic variables, and by measuring these responses, these derivatives can also be worked out. This calls for establishing the formal relationship between these dynamic responses and the aerodynamic derivatives, thus leading to aerodynamic modeling.

The pilot will maneuver an aircraft using the pitch stick, roll stick, and rudder pedals besides using the throttle lever arm. The movements of these devices forward, backward, or sideways are transmitted via the control actuators to the moving/hinged surfaces of the aircraft. These surfaces are extensions of the main surfaces, like wing, rudder fin, etc., and are called control surfaces. The movements of the control surfaces interact with the flow field while the aircraft is in motion and alter the force and moment balance (equations), thereby imparting the ‘‘changed’’ motion to the aircraft until the new balance is achieved. During this changing motion (which can be called perturbation), the aircraft attains new altitude, acquires new orientation, etc., depending on the energy exchanges and balance between kinetic, potential, and propulsive (thermal) energies. At microscopic level (or scale) this behavior can be studied by exploring the effect of the aerodynamic param­eters discussed in the following section. At the macroscopic level (or scale) the behavior can be studied using the simplified EOM and resultant transfer function (TF) analysis. Hence, we gradually get closer to flight motion and hence flight mechanics.