Possibility of Loss of Blade Flapping Motion Stability

The main rotor hubs in which the angle between the horizontal hinge axis and the radial line is 90°, i. e. , 6 = 90°, have a serious problem: loss of

flapping motion stability. By loss of flapping motion stability, we mean possible deflection of the blade upward or downward to the horizontal hinge stops. This phenomenon takes place as a result of variation of the blade incidence angles during flapping, together with the presence of a blade lag angle.

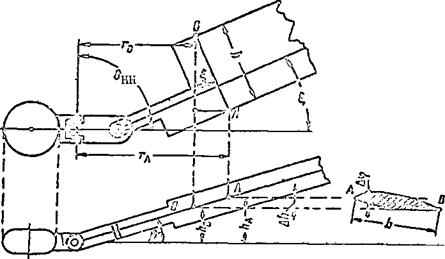
If the blade rotates relative to the vertical hinge through the lag angle then the blade element chord AB will not be parallel to the horizontal hinge axis. During flapping motions, the leading edge and trailing edge of the blade element will displace along two different radii: the point A at the

leading edge will have the larger radius r, the point В on the trailing edge

A

will have the radius r„ (Figure 50).

D

When the blade flaps upward through a certain flapping angle B, the points A and В move up different distances relative to the main rotor hub rotation plane. Point A will move to the height h^, while point В moves to the height hg. As a result of this height difference, the additional angle Да develops /69

between the blade element chord and the hub rotation plane. The larger the blade lag angle and the larger the change of the flapping angle, the larger the incidence angle increase will be.

We see from the figure that

where b is the blade element chord length.

Figure 50. Blade pitch change during flapping motions.

The increased height of the leading edge above the trailing edge Ah is found from the formula

Д/г — a tg A£,

where a = b sin £ is the projection of the chord on the radial line. Consequently

i. e., the previously drawn conclusion is confirmed.

If there is a lag angle £, the blade element incidence angle will increase during up-flapping and will decrease during down-flapping. This variation leads to increase of the blade thrust and its moment relative to the horizontal hinge during upward flapping, i. e., the blade will travel up against the stop.

During down-flapping of the blade, blade thrust will decrease still further, which leads to downward travel of the blade against the lower stop.

This is then the manifestation of the loss of flapping motion stability.

How can these undesirable phenomena be eliminated? The simplest technique is to increase the degree of pitch horn compensation, i. e., increase the com­pensation coefficient. However, increase of this coefficient leads to an increase of a particular type of main rotor blade vibration — a vibration of the flutter type. Therefore, the loss of flapping motion stability is elimi­nated at the present time by a different approach — a change of the basic geometry of the main rotor hub.

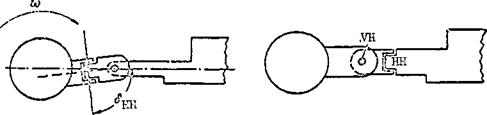
To accomplish this, a hub is used in which the angle between the horizon­tal hinge axis and the longitudinal blade axis with the blade in the radial

position is less than 90°, i. e., 6 < 90° (Figure 51). Such a hub is

Ші

installed, for example, on the Mi-1 helicopter.

If the blade of such a hub is rotated through the lag angle E, = 90° – 6^ its longitudinal axis is then perpendicular to the horizontal hinge axis.

This means that the radii of rotation about the horizontal hinge for the /70

leading and trailing edges approach one another, i. e., r = rg (see Figure 50).

In this case, flapping motions will not lead to any height increment Ah.

Therefore, there will not be any increase of the incidence angle Ah, and the flapping motions remain stable.

If the lag angle £ 51 90° – 6 , the incidence angles vary just as for

HH

= 90°. However, in this case, the instability of the flapping motions ШІ

Figure 52. Schematic of main rotor hub.

Figure 51. Schematic of main rotor hub.

shows up to a lesser degree.

However, if the vertical and horizontal hinges of the main rotor are located in the reverse order, the factors which cause loss of flapping motion stability can be completely eliminated (Figure 52).

With this hinge arrangement, rotation of the blade about the vertical hinge does not cause any change of the position of the blade element chord relative to the horizontal hinge axis.

Consequently, for this hub the radii of rotation of the leading edge and

trailing edges about the horizontal hinge axis will always be the same,

(r. = r„) , i. e., there will not be any change of pitch during flapping motions, A 15

and loss of flapping motion stability will not occur.