Measurement of the coefficient of heat transfer by convection

A non-dimensional expression of the film coefficient h [/m-2s-1] should be used, for example the Nusselt number (Nux = hx/l) or the Stanton number (St = Nux/RexPr), because numbers can be conveniently expressed in terms of other characteristic numbers. For example, for a flat plate in a gas stream (Pr и 1):

Nux = 0.332(Rex) (Pr) laminar boundary layer

Nux = 0.0296(Rex) (Pr) turbulent boundary layer

By the change of Nusselt number with the abscissa, the transition from laminar to turbulent regime can be determined. The measurement of h can be made with an unsteady method.

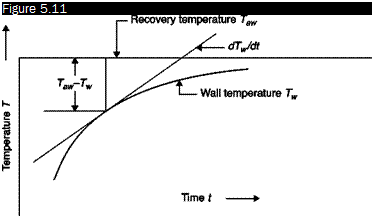
In a hypersonic flow, a body, which initially is at room temperature, undergoes aerodynamic heating, the temperature rises very quickly at the beginning then more and more slowly as the difference between the wall temperature and the adiabatic (or recovery) wall temperature decreases. If the model is made with an insulating material, the temperature of each point of the model tends asymptotically to the value of the local adiabatic wall temperature (Figure 5.11).

If the wall temperature is measured with a thermocouple using the technique in Figure 5.2, with the wall made of an insulating material, it can be assumed that all the energy exchanged by convection through the metal disk of area A goes to increase the internal energy of the metal plate to which the thermocouple is welded. The coefficient of heat transfer by convection, h, can be obtained from the unsteady energy balance:

qA = hA AT aw – Tw) = mcd – h= me dTwldt

A Taw – Tw

Determination of the coefficient of heat transfer by convection with the unsteady method

where m is the mass of the disk, c the specific heat of the wall and dTJdt the slope of a plot Tw (t) obtained in the measurement (Figure 5.11).

When the flow is not hypersonic, this technique can be still applied, up to Mach numbers close to zero, by a prior heating or cooling of the body with respect to the stream temperature. For M ^ 0, static temperature coincides both with the stagnation temperature and with the adiabatic wall temperature.