Adjoint Green’s Function for a Conical Surface

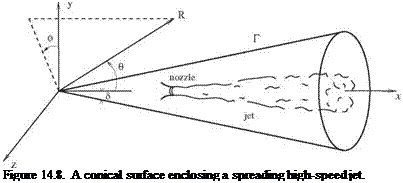
The noise of high-speed jets is an important aeroacoustic problem. Because the mixing of the jet and ambient gases, the jet expands laterally in the downstream direction. The use of a cylindrical surface Г for this type of flows would not be appropriate. A conical surface with a well-chosen half-angle 5 is a good choice. Figure 14.8 shows a conical surface Г enclosing a high-speed spreading jet. The natural coordinates to use outside the conical surface is the spherical polar coordinates (R,0,Ф).

Let p(g)(R,0,ф, t; R0^0, t0) be the direct surface Green’s function with the source point located at (R0, ф0) and source time t0. The Fourier transform of time t is

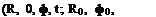
(14.60)

The far-field sound pressure due to a surface pressure distribution of Ф (R0, ф0, t0) on the conical surface is then given by

p(R, 0, ф, t) =

to 2n to to

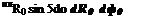//// pig)(R, 0, ф; R0, ф0; o; Ц)Ф^0, ф0, t0)e

—TO 0 0 —TO

The surface element of a conical surface is dS = R sin SdRd(p. By the reciprocity relation of Eq. (14.52), p(g) is related to the adjoint Green’s function v’f’1 by

p®(R1> $1, ф1; R0, ф0; t0) = v^ (R0, ф0; R1, в1, ф1; to), (14.62)

where v(n) is the adjoint velocity component normal to the conical surface Г. Positive is in the outward pointing direction.

As for the case of a cylindrical surface, a particular solution of the adjoint equations is given by Eq. (14.55). The relationship between cylindrical coordinates and spherical coordinates is

r = R sin в, x = R cos в.

On replacing r and x by this relation and upon using Eq. (14.55), the particular solution (denoted by a subscript “p”) may be written as

СО / ч

p(a) = -2 Д (R1-R cos в cos e1 Jm 2R sin в sin в1 e-im 2 +іт(ф1-ф).

Pp 8n2fl0R1 m=—О ma0 V

(14.63)

The adjoint velocity is related to the pressure by Eq. (14.49). Thus, the compo­nent normal to and on the conical surface is

Now, let the homogeneous solution (denoted by a subscript “h”) of Eq. (14.49) and (14.50) in cylindrical coordinates to have the following form:

This is a Fourier series expansion in angular variable (ф — ф1). The governing equations for the amplitude functions (u(m, vJ, wJ, pm ) can readily be found by substituting Eq. (14.65) into Eqs. (14.49) and (14.50) with the nonhomogeneous term omitted. These equations are as follows:

• ‘• (a) 29pm n mum a0 =0 d x		(14.66)
■ Ha) 29pm n i0JVm a0 d r = 0		(14.67)
— i^w> m + ial = 0		(14.68)
d v(a) v(a) m л(а) dvm vm. .m ~ (a) ію pm « + i w(m d r r r m	d u(a) °um 0 dx ’	(14.69)

—i – ю R cos 5 cos 0

The boundary condition from Eq. (14.51) is

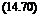0 = 5, pm = – Jm[ —R sin 5 sin 01 ) e

a0

For future reference, it is easy to show that by taking the complex conjugate of Eq. (14.66) to Eq. (14.70) that

u—m (r, x; 01, —ю) = am* (r, x; 01, ю) (14.71)

v(—m (r, x; 01, —ю) = v(m’ (r, x; 01, ю), (14.72)

where * denotes the complex conjugate.

Once u(m) and v(m) are found, it is straightforward to find, by combining with par­ticular solution Eq. (14.64), that the normal velocity of the adjoint Green’s function on conical surface 0 = 5 with (R, ф) replacing by (R0, 00) is

mm (R0, ф0; R1, 01, ф1; ^0,ы)

. I UJ

+ i cos 01 sin 5Jm I —R0 sin 01 sin 5

a0

— i [v(m)(R0, 5; ю) cos 5 — , 5; ю) sin 5]} ■ e—imп +т(ф1— ф0). (14.73)

By means of the reciprocity relation (14.62), the pressure associated with the direct Green’s function is now known, i. e.,

For later application, it is useful to expand p(g) as a Fourier series in (ф — ф0) in the following form:

Figure 14.9. An oblique Cartesian coor­dinate system for computing the homo­geneous adjoint Green’s function.

By means of Eqs. (14.74) and (14.73), it is easy to find that

+ i cos в sin SJm sin в sin SR0 e a" 0

a0

v-)(R0S, o) cos S – ui‘ma)(R0tS, o) sin sJJ. (14.76)

% R

Note: The dependence of pm on R is in the factor. An important property of pm (R, в; R0, o) is that it becomes its complex conjugate if m ^ -m and о ^ – o, i. e.,

p-m (Яв; R0> -0) = Pm (R^; R0,o>.