Aircraft equations

An aircraft, in contrast to a missile, does not have all of its principle axes aligned with the body axes. The 1B axis may be parallel to what is called the water line (an expression borrowed from naval architecture), aligned with the zero-lift attitude, or just poke through the nose of the radome. Whatever the case may be, it is not, in general, the principal MOI axes, nor is the 3B axis. However, thanks to the planar symmetry wrt the Ій and 3B axes, the 2B axis is indeed a principal axis. Such a configuration gives rise to a MOI tensor in body coordinates of the form

In 0 /13

0 /2 0

/31 0 /33

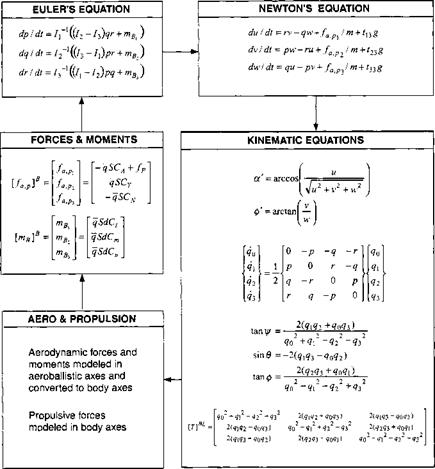
Fig. 10.1 Summary of kinematic and dynamic equations of missiles.

with product of inertia components at the 1,3 and 3,1 locations and /в = /31. On the diagonal we have the axial moments of inertia /ц and /33 and the principal moment of inertia /2.

We can program Euler’s equation (10.10) directly, tolerating the inversion of the moment of inertia matrix, or, because there are still four zero elements in [IB]B, it is possible to solve for the body rate components explicitly and write down the scalar equations.

Before proceeding, I want to expand the angular momentum vector with addi­tional rotary devices like propellers or turbojets, which are an important feature of airplanes. Let the rotary frame be BR and its c. m. BR. The angular momentum is designated Ibbrre and the angular momentum of the remaining rigid aircraft cell is as previously lBBE = ІвшВЕ. Combining both according to Example 6.6, the total

MOI referred to the common c. m. C becomes

‘YaBE ____ jBE і jBr,

c — lB lB

and Euler’s law

DElf + DElBBf= mc

As before, we transform the rotational derivative to the reference frame associated with the aircraft cell В:

DBlf + ПВЕ1ВВЕ + DBlBBf + nBElB/RE = mc

Now, let us simplify the solution by neglecting changes in rotary motion DBlBBE = 0, i. e., assuming that the propellers or turbojets spin at constant speed. With the additional stipulation that the aerodynamic and propulsive moments can be referred to the cell’s c. m. В (rather than C), we obtain the tensor equation for the attitude motions, without incurring any significant errors

IBDBu, BE + Пве(івшве+і%Е) = mB

Expressed in aircraft cell coordinates ]B

and solved for the body-rate derivative

Compare this equation with Eq. (10.10) and note the coupling of the rotary angular momentum [1Brr e]B with the aircraft body rates [Q. BE]B. This vector product models the gyroscopic effect that the rotary engine imparts on the aircraft.

Before we express the component equations, let us make the reasonable assump­tion that the spin axes of all rotary parts are parallel to the aircraft longitudinal axes; therefore, [lBRRE]B = Ur 0 0]. Now with In = hi, we express Eq. (10.17) in coordinates

dp/dt	‘ (	‘hi	0	Лз	Л	/	0	—г	q
dq/dt	=	0	h	0		–	г	0	-р
dr/dt	l	7із	0	/33	)	V	_~q	Р	0

/

~hi

0

Лз

В

р

В

Ir

Б

тВ]

Б

X

0

h

0

q

+

0

+

тВг

V

Лз

0

Лз

г

0

У

тВз

/

derivatives is somewhat convoluted. I will spare you the details and just provide the result:

As you can see, the scalar equations of motions of an aircraft are much more complicated than those for missiles, Eq. (10.16). You have a choice now, either to program these scalar equations or the matrix equations (10.17). With today’s computer power at you fingertips, I recommend you use the simpler, less error prone matrix formulation.

Comparing Eqs. (10.16) and (10.18) provides us with some insight into the differences of missiles and aircraft dynamics. Let us first contrast the roll equations. The product of inertia Icouples the pitch and yaw rates to the roll degrees of freedom of the aircraft. The greater its value, the stronger the coupling. If 713 = 0, the missile roll equation is recovered. The pitch and yaw equations exhibit similar trends. Other phenomena caused by A 3 are the coupling of the aerodynamic yawing moment m Bj into the roll axis and the aerodynamic rolling moment m Bl into the yaw axis. Finally, the rotary angular momentum Ir couples through the pitch rate into both the roll and yaw derivative equations, while the yaw rate connects it with the pitch derivative.

Could we have drawn the same conclusions from the matrix equation (10.17)? The answer is yes, in a general sense; it shows the coupling of the body rates IcoBE]B through the MOI tensor [IB]B into the body-rate derivatives, as well as the coupling of the aerodynamic moments [mB]B through the inverse ([Ig ]B)_1. Furthermore, the vector product of [ QDE |B couples the rotary angular momentum [lBlk]B. However, by comparing Eqs. (10.17) with (10.10) we would not have discovered the much simpler missile equations, unless we had scrutinized the simplifying effect of the diagonal moment of inertia matrix.

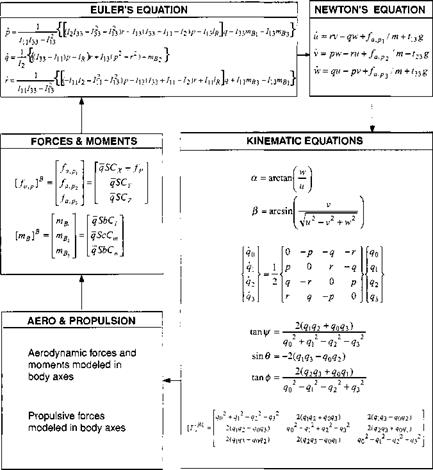
To sum up, I have assembled the important aircraft equations in Fig. 10.2, just as I did with the missile equations in Fig. 10.1. Euler’s equations are copied from Eq. (10.18), and Newton’s equations are adopted unchanged from the missile model. The incidence angles are now angle of attack a and sideslip angle /3, and the aerodynamic forces are modeled in body axes directly.

The missile and aircraft six-DoF equations are the bread and butter of simulation engineers. They were derived with the assumption that the Earth is the inertial frame and that the curved Earth can be unfurled into a flat plane. How these equations are integrated with other subsystems, such as autopilots, actuators and navigation systems will be described later. Before we leave this section, however, I would like

Fig. 10.2 Summary of kinematic and dynamic equations of aircraft.

to derive the especially interesting equations of motions for spinning missiles and Magnus rotors.