Category Aircraft Flight

If we change the wing area of the aircraft while keeping the weight constant we change its wing loading (aircraft weight/wing area). The effect of this is shown in Fig. 7.5 where it can be seen that the result of an increase in wing loading is to move the drag curve to the right of the picture without altering the drag values.

The explanation of this is quite simple. At any point, A, in Fig. 7.5 the lift (equal to the weight of the aircraft) is given (Chapter 1) by the lift coefficient multiplied by the wing area and the dynamic pressure (-pV2). Assume that we reduce the wing area. If only the size of the wing is changed but its geometrical shape and angle of attack remain the same, the lift coefficient will be unaltered. We can then obtain the same lift force by increasing the speed to raise the dynamic pressure, so compensating for the area reduction.

The drag coefficient will also be unchanged for our smaller wing. Therefore, since the product of dynamic pressure and wing area is unaltered, the drag force will also be unchanged. Point A is therefore simply moved horizontally to A’ (Fig. 7.5) and the entire curve is shifted as shown and somewhat spread out, the minimum drag retaining the same value as before.

At this point it is worth pointing out that our argument is somewhat approximate. Unless the fuselage and tail assembly are scaled in the same way as the wing, the drag coefficient will be changed as we go from point A to point A’ (Fig. 7.5). Thus some change in the minimum drag value will be obtained.

A further factor we have ignored is that a change in the size of the wing will also require a change in the weight of the structure, so our assumption of constant weight is questionable, particularly if we consider large changes in wing area.

However, it remains generally true that increasing the wing loading means that the aircraft can fly faster with little penalty in terms of increased drag. This has led to an increase in wing loadings for many aircraft types, and this is further described in Chapter 9. It must be remembered that any such increase in wing loading will mean a higher minimum flying speed, and so a com­promise must be reached between requirements for cruise and landing and take-off performance.

Hypersonic flight using a scramjet engine has been something of an aero­nautical engineer’s dream ever since the late 1960s. The American National Aerospace Plane (NASP) programme involved building a prototype, the X-30 research aircraft. However after some 2.4 bn dollars was spent of the project,

it was abandoned in 1994. This project was then followed by a lower cost approach involving the use of an unmanned 12 ft long vehicle, the X-43, shown in Fig. 8.24. This was mounted on the nose of a Pegasus booster rocket, and the combination was launched from under the wing of a B52 bomber. After a major failure in the first flight, two later successful flights were made, with the X-43A finally reaching a maximum speed of Mach 9.68 in 2004, after a fuel burn of some 11 seconds. The viability, though perhaps not yet the financial practicability, of scramjet-power hypersonic flight was thus established.

Recommended further reading

Kdchemann, D., The aerodynamic design of aircraft, Pergamon Press, 1978, ISBN 0080205143. A masterpiece from the ‘Father of Concorde’ which stands the test of time. Peebles, C., Road to Mach 10: Lessons learned from the X-43A flight research program, AIAA, Reston VA, USA, 2008, ISBN 9781563479282. A fascinating and detailed description of the X-43 project.

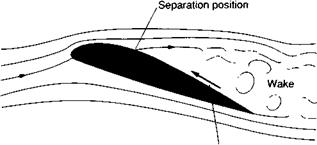
Flight in the range between the onset of important compressibility effects (M = 0.7) and the establishment of fully supersonic flight conditions on the other side of the drag coefficient rise (M = 1.4) is said to be transonic. The tran­sonic range poses some of the most difficult problems for the aerodynamicist but it is of great practical importance. Not only do supersonic aircraft need to have satisfactory characteristics to accelerate and decelerate safely through the transonic range but currently many aircraft are designed to cruise close to the speed of sound.

The reason for this has been given in previous chapters. Because the efficiency of a gas turbine engine increases with design speed, we wish to fly fast. How­ever, as the speed of the aircraft approaches the speed of sound a sudden rise in drag occurs, together with other problems such as the production of sonic bangs on the ground. For most transport aircraft, and a number of military air­craft designed for such roles as ground attack, a suitable solution is obtained by restricting the cruising speed to just below the drag-rise Mach number.

In Chapter 5 we saw how compressibility effects and shock waves put up the drag as the speed of sound is approached. For a given aircraft the typical vari­ation of drag with Mach number is shown in Fig. 5.19. The very rapid increase in drag coefficient near the speed of sound is clearly shown. Some wing sections also produce a slight dip in drag immediately before the rise. Figure 9.1 shows this effect. It is caused by the rise in lift coefficient in Fig. 5.19 which offsets the smaller rise in drag coefficient initially for a wing operating at constant lift rather than constant angle of attack.

At first sight it might seem to be best from the point of view of obtaining economical cruise conditions to keep well below the drag rise Mach number. However as we saw in Chapter 3, the efficiency of the gas turbine rises with Mach number and it is therefore worth pushing the cruising speed as close to the speed of sound as possible to obtain the best compromise between airframe and engine performance. It may also be worth exploiting the drag coefficient ‘dip’, mentioned above, at the same time.

These factors have resulted in the development of a whole series of airliners cruising at a speed just below that at which the transonic drag rise occurs. This has had the added advantage of providing the travelling public with high speed transportation over a wide variety of distances – and one of the most appeal­ing features of air transport has always been speed.

In this chapter we will consider the aerodynamic development of aircraft designed for flight at transonic speeds, with particular emphasis on the prob­lems associated with transport aircraft. However it must not be forgotten that many military aircraft, such as ground attack aircraft (Fig. 9.2) are also designed primarily for transonic operation and some reference will also be made to these where appropriate.

The development of civilian transport aircraft over a period of some 30 years is illustrated in Fig. 9.3 in which two aircraft are shown spanning the period from the earliest jet transport, the de Havilland Comet, to a much later design, the Airbus A340. An intermediate development, the Trident is shown in Fig. 3.9. In some ways the three configurations look remarkably similar, the main obvious development being the introduction of pylon mounted engines rather than the buried installation of the Comet. Closer examination, however, reveals other changes. Firstly there has been a reduction in the plan – form area of the wing for a given aircraft weight; in other words an increase in the wing loading. Next there has been a tendency for the sweep angle firstly to increase but, surprisingly to be reduced in the more modern designs. Close examination of the aircraft themselves would also reveal considerable differ­ences in the wing sections used.

The choice of sweep angle is a question of a compromise between using enough sweep to reduce the effects of compressibility, as will be explained below, and avoiding the unpleasant low speed handling effects (Chapter 2).

The reason for wishing to use high wing loading simply comes from the need to reduce area for a given weight, and the need for this was discussed in Chapter 8.

As we have seen previously, all aircraft design is a compromise, and the actual minimum area may well be dictated by landing requirements rather than cruise requirements. The increase in wing loading thus owes a great deal to the work of the low speed specialist in producing ever more sophisticated and effective high lift devices for use during take-off and landing. Some of these have been described in Chapter 3, and this is likely to be a major field of aero­dynamic research and development for some considerable time.

Even the term ‘low speed specialist’ used in the above paragraph must be treated with some caution. The aircraft may itself be flying at low speed in the sense that the speed of flight is well below the speed of sound. However, extremely low pressures may well be developed over the upper surface of such devices as leading edge slats, and what at first sight may appear to be a low speed flow, may well contain localised regions in which the flow is near to, or even exceeds the speed of sound.

After this slight digression into the necessary problem of ‘off design’ perform­ance, we now return to the problem of designing a wing with good performance at the cruise condition. At this stage it is worth emphasising that, for the type of aircraft we are considering, the cruising speed will be just below the speed of

sound, in order to avoid the full effects of the transonic drag already described. The basic problem is therefore to try to push the wing loading as high as possible, while at the same time delaying the onset of this drag rise to as high a Mach number as possible. The requirement for high-wing loading implies low local pressures on the upper surface of the wing, and consequently high local speeds. These high speeds, however, are the very thing that is likely to lead to the formation of shock waves which cause the transonic drag rise. It is to the problem of resolving this dilemma that we now turn our attention.

For aircraft designed for cruise in the transonic range the use of swept forward, rather than swept back, wings offers some advantages. An optimum spanwise load distribution can be obtained with conventional taper towards the tip. The problem of boundary layer drift towards the tip, which encourages tip stall is also alleviated. Because the velocity component along the leading edge is now directed inboard, the boundary layer tends to thicken towards the root rather than the tip.

With this catalogue of virtues the reader may wonder why forward sweep has not been employed exclusively. The main problem lies with the struc­tural behaviour of the wing. When the wing is loaded the angle of attack increases unlike the swept back wing (Fig. 9.13). Because of this the tip lift is increased and the deflection worsens. The wing can then suffer progressively increasing twist. This condition is known as divergence and is encountered again in Chapter 14. The problem is made worse because an increase in load at the tip of a swept forward wing will produce a nose-up pitching moment thus increasing the angle of attack over the whole wing and again increasing the load.

After early attempts at using forward sweep (e. g. the Junkers 287 in 1942) the structural problems led to the virtual disappearance of the idea. Recently, however, advances in structural materials have led to renewed interest in the concept. Modern composite materials (Chapter 14) allow suitable flexural behaviour to be designed into the wing to prevent the occurrence of divergence. Another technique which can be employed is to automatically sense the twist as it occurs and to use a computer-driven system to deflect the ailerons down­ward simultaneously in order to cancel the twisting effect.

The X-29 aircraft (Fig. 9.20) is an example of an experimental forward – swept configuration. Radical new design features, such as forward sweep, are, however, expensive and commercially risky to introduce. It is likely to be many years, therefore, before the conventional swept back configuration is seriously challenged.

In the discussions above, we have assumed that the control stick is being held firmly, so that there is no movement of the control surfaces. However in real­ity, changes in aircraft attitude will alter the loading on the control surfaces; trying to move them.

Some power-operated controls are irreversible; that is, the aerodynamic loads cannot drive them. On most aircraft, however, and invariably on those with servo-assisted or manual controls, the elevators will move in response to changes in pitch, if the stick is not held firmly. The influence on stability of allowing the stick to move freely depends on the design of the surfaces and the control mechanism. The degree of aerodynamic balancing, the stiffness and the inertia of the system components are all important factors. In general the effect of leaving the stick free is to reduce the static stability.

Because of the very real difficulty and high pilot workload during landing this phase of flight has been the subject of rapid development in systems to aid the pilot in his task. As well as improving safety these systems allow for better aircraft utilisation because one of the obvious limitations for operation under

This wing low

Compensating rudder

causing drift

Sideslip relative to wind

Fig. 13.10 Use of sideslip to correct drift

Aircraft is rolled slightly to induce sideslip into wind Aircraft axis is kept aligned with runway by use of rudder

purely ‘visual flight’ is that low cloud may make the approach impossible even to attempt.

Here we shall very briefly describe some of these aids, the proper study of which is a separate discipline in its own right.

It is apparent from the above discussion that one of the main problems in landing is that of following an accurate glide path to the runway threshold. This can, of course, be particularly difficult in conditions of poor visibility par­ticularly for large aircraft where the glide path needs to be established several miles from the touch-down point. The main purpose of any landing aid is thus to aid accurate flying during this phase of the landing. For modern aircraft a number of such aids is available. Among these are radio beacons which can be used for general navigational purposes as well as landing aids such as the non-directional beacon (NDB) which supplies the aircraft with a directional ‘fix’ on a known ground location or the very high frequency omni-directional beacon (VOR) which supplies both directional and range information. The most common aid dedicated solely to landing is the Instrument Landing System (ILS). In this a pair of radio beams are arranged to cross on the glide path. Deviation from the glide path is then indicated by a cockpit instrument, the ILS indicator (Fig. 10.2). Satellite-based GPS systems are also used.

Automatic flight along the glide path can be achieved by adding an auto­matic throttle and flight control system, with accurate height information being obtained from a radio altimeter. Automatic flare is provided to bring the air­craft on to the runway. Fully automatic landing systems of this type have greatly increased the range of conditions under which safe aircraft operation is possible.

A newer alternative to the ILS system is the more accurate Microwave Landing System (MLS). This system is however being challenged by an even newer technology, the Global Navigation Satellite System (GNSS). The advan­tage of the latter is the that it relies on signals from satellites and does not require expensive ground installations at airports. After lengthy trials, a satel­lite landing system has been cleared for use at a small number of selected airfields.

For most wing sections, the amount of lift generated is directly proportional to the angle of attack, for small angles; the graph of CL against angle of attack is a straight line, as shown in Fig. 1.17. However, as illustrated, a point is reached where the lift starts to fall off. This effect is known as stalling. The fall-off may occur quite sharply, as in Fig. 1.17 which shows the variation of lift coefficient with angle of attack for a wing with a moderately thick aerofoil section (15 per

cent thickness to chord ratio). You can see that the stall occurs at an angle of attack of around 12°. A thin uncambered wing may stall even more sharply, and at an angle of attack of 10° or less. A sudden loss in lift can obviously have disastrous consequences, particularly if it happens without warning.

The stalling characteristics of an aircraft wing depend not only on the aero­foil section shape, but also on the wing geometry, since not all of the wing will stall at the same angle of attack.

Stalling occurs when the air flow fails to follow the contours of the aerofoil and becomes separated, as illustrated in Figs 1.18 and 1.19. The causes of this flow separation are dealt with in detail in Chapter 3.

Once the flow separates, the leading-edge suction and associated tangential force component are almost completely lost. Therefore, the resultant force due to pressure does act more or less at right angles to the surface, so there is a significant rearward drag component. The onset of stall is thus accompanied by an increase in drag. Unless the thrust is increased to compensate, the aircraft will slow down, further reducing the lifting ability of the wing.

After the stall has occurred, it may be necessary to reduce the angle of attack to well below the original stalling angle, before the lift is fully restored. An aircraft may lose a considerable amount of height in the process of recovering from a stall, and trying to prevent its unscheduled occurrence is a major con­cern of both pilots and aircraft designers. Later on, we shall describe some of the preventive measures and warning systems that may be employed.

Apart from the problem of wing stalling, there are several areas in the flow where we wish to prevent flow separation, or inhibit the build-up of thick low-energy boundary layers. Flow separation in air intakes of gas turbine engines is a particularly serious problem, since it is most likely to occur at high angles of attack on landing and take-off; just the time when it is least wanted. Stalling of the intake flow can cause the engine to lose power, or flame-out (switch-off) altogether, with potentially disastrous consequences. Some air­craft are even fitted with a device that automatically operates the starting igniters at high angles of attack.

In high speed flight, flow separations may also be caused by the interaction between shock waves and a thick boundary layer. Notice how the air intake of the supersonic Tornado shown in Fig. 3.15 is separated from the fuselage, to form a slot through which the fuselage boundary layer can pass, preventing its interfering with the intake flow.

In addition to the problem of air intakes, it is also important to prevent separation in the vicinity of control surfaces, since the last thing we want to lose in the approach to a stall, is the ability to control the aircraft.

One way to prevent local flow separation is to apply engine generated suction via small slots or openings in sensitive areas. An alternative pass­ive measure is the attachment of small tooth-like vortex generators on the surface. These are designed to give a highly turbulent surface flow, thus inhibiting separation. Figure 3.7 shows the vortex generators on the wing of

a Buccaneer. This type of vortex generator may be seen on many early swept – wing aircraft, where they were used to try to overcome the problems described below.

We have spent some time in looking at the differences between flows at high and low speed. It is worth emphasising that, for both the duct flow and the ‘external flow’, although Bernoulli’s equation becomes inaccurate as speed increases, it is still true that an increase in speed is accompanied by a decrease in pressure, irrespective of whether the flow is sub – or supersonic.

We find that our criterion for high speed, introduced above (the speed at which density changes first become apparent) is related to the Mach number. For an aircraft this usually occurs at flight Mach numbers above about 0.5. Rather than a single measure of what constitutes a ‘high speed’ we can now begin to identify Mach numbers at which distinguishing features of high speed flow begin to appear (Fig. 5.5).

This figure shows the Mach numbers at which we will obtain our typical low subsonic and fully developed supersonic flows. It also shows a number of other features, which we will discuss shortly, such as the intermediate stage between these flows, the transonic speed range. The advent of important heating effects caused by the passage of the aircraft through the air is also shown.

The overall efficiency of a gas turbine propulsion system depends on two major contributions, the Froude efficiency which, you may remember, is related to the rate at which energy is expended in creating a slipstrean or jet, and a thermal efficiency, which is related to the rate at which energy is wasted by creating hot exhaust gases.

As noted earlier, the Froude propulsive efficiency of the pure turbo-jet is low, because thrust is produced by giving a small mass of air a large change in velocity. However, for a fixed amount of thrust, as the speed of a jet or gas – turbine-propelled aircraft increases, the air (mass) flow rate through the engine also increases. A smaller change in velocity is needed for this larger mass of air, and the Froude efficiency thus improves. However, for an aircraft in steady level flight, the thrust required is equal to the drag. Since the drag varies with speed, the thrust required must similarly vary, so the overall efficiency of propulsion depends on the drag characteristics of the aircraft. This inter­dependence between the propulsion device and the aircraft aerodynamics is an important feature of aircraft flight and is described further in Chapter 7.

In general we may wish to design for one of two goals as far as the climbing performance is concerned. Firstly the climb angle rather than the rate of climb may be of primary consideration. This will be true, for example, if we are con­cerned with the take-off performance. The primary concern will be to avoid hitting high structures in the vicinity of the airport and for this it is the angle of climb that is critical. In other circumstances it may be the rate of climb that is the factor of most interest. This would be true, for example, for an interceptor aircraft. It is important at this stage to realise that the maximum angle of climb and the maximum rate of climb do not occur together, but as we shall see, at two distinct operating points.