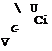The main rotor blade element may be compared with an airplane wing element. Let us examine the aerodynamic forces acting on an airplane wing and then transfer these forces to the blade. The flight force Y and the drag force X develop on the airplane wing at the angle of attack a. The geometric sum of these forces will be the resultant aerodynamic force R = Y + X. The angle between the lift force Y and the resultant aerodynamic force R vectors is called the aerodynamic efficiency angle (0 ). The larger the aerodynamic efficiency angle, the lower the aerodynamic efficiency of the wing, since the minimal aerodynamic efficiency angle corresponds to maximal wing aerodynamic efficiency ctg 0 =
= Y/X = K. Reduction of the aerodynamic efficiency angle means a sort of "attraction" of the resultant aerodynamic force vector AR to the lift force vector Y, i. e., it means reduction of the backward tilt of AR relative to the normal to the undisturbed flow.
|
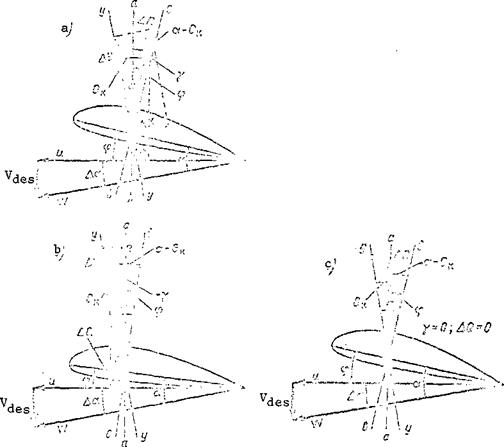
Figure 75. Blade element autorotati-ve conditions.
|
Now let us turn to examination of the forces acting on the blade. We draw three straight lines through the center of pressure of the blade element: a-а is perpendicular to the rotor rotation plane; b-Ъ is perpendicular to the blade element chord; y-y is perpendicular to the resultant velocity vector (Figure 75a). The angle between the lines y-y and b-b and the angle a are equal, since they are formed by mutually perpendicular sides. On this same basis, the angle between the lines a-a and b-Ъ is equal to the incidence angle ф.
The resultant aerodynamic force vector is applied at the blade element
center of pressure. We resolve this force into the lift force AY and the drag
force ДХ. The angle between the vectors AY and AR is the aerodynamic efficiency
angle The angle between the vector AR and the line b-b will be equal to
the difference of the angles (a – 0 ) . If this difference is less than the
blade incidence angle, then ф – (ot – 0 ) = у, i. e. , the angle у is positive. This
K.
means that the projection AQ of the force AR on the main rotor plane of rotation will be directed aft and creates a retarding moment which reduces the rotor rpm.
The main rotor will operate in the decelerated autorotative regime, which leads to stopping of the rotor. The larger the blade element incidence angle or pitch, the larger the angle y, and the larger the force AQ and its decelerating moment.
If the difference between the angle of attack and the aerodynamic efficiency angle is greater than the incidence angle, i. e., (a – 0 ) > ф, then
К
Ф – (a – 0„) = – y. The angle у is negative, which means that the vector AR /121
K.
is directed forward relative to the hub axis (Figure 75b). The projection AQ of the force AR on the hub rotation plane is directed forward and creates a turning moment which accelerates the rotor rotation. The main rotor will operate in the accelerated autorotative regime. The smaller the incidence angle ф, the larger the forward tilt of the vector AR, and the higher the speed at which the rotor turns.
If the difference (a – 0 ), then ф – (a – 0 ) = 0, i. e., the force AR is
К к
parallel to the hub axis and its projection on the hub rotation plane AQ = 0 (Figure 75c). In this case, the retarding or turning moment equals zero, and the rotor revolves at constant rpm, i. e., the rotor autorotative regime will be established.
From these examples we conclude:
— the tilt of the elemental resultant aerodynamic force vector depends on the blade element pitch;
— with reduction of the pitch, the force vector AR is deflected forward, and the main rotor autorotation becomes accelerated;
— with increase of the blade element pitch, the force vector AR is deflected aft, and the main rotor autorotation becomes decelerated.
The dependence of the autorotative regime on the blade element angle of
attack and pitch can be expressed graphically (Figure 76). This graph is
called the autorotation margin graph. The abscissa is the blade element
angle of attack, the ordinates are the incidence angles ф and the angles equal
to the difference a – 6 .
К
Let us examine the characteristic points in this figure. The ascending portion of this curve corresponds to blade element angles of attack below stall. The point В is the stall angle, and the descending portion corresponds to angles above stall.
If we draw a straight line parallel to the abscissa axis, it crosses the curve at the two points A and C.
In the figure, this straight line passes through the point on the ordinate corresponding to the incidence angle ф = 4° (such a straight line can be drawn through any point of the ordinate). What do the points A and C characterize? The point A corresponds to the blade element angle of attack (in our example a = 7°30′) which corresponds to steady state autorotation. Let us show that this actually is the case.
It was established above that steady state autorotation will occur when
the difference a – 0 = ф. In this case, the force AR of the element will be
К
parallel to the main rotor hub axis. In our example ф = 4° and а – 0^ = 4°. 180
This means that y= 0 (see Figure 75c). Therefore, in order for the Mi-1 helicopter blade to have steady state autorotation with ф = 4° the blade angle of attack must equal 7° 30′. Points of the curve for which Ct-0 <4 correspond to smaller angles of attack, i. e., the autorotation will be decelerated (see Figure 75a). At angles of attack between the points A and C all the points of the curve correspond to the inequality а-0^>ф, i. e., accelerated autorotation (see Figure 75b). But at angles of attack up to the stall angle, the accelerated autorotation will be stable, while at angles above stall flow separation takes place, and the autorotation is unstable.
The range of angles of attack between the points A and C is called the blade element autorotation margin. Since flight with blade element angles of attack above the stall angle is not feasible in practice, the autorotation margin will correspond to the angles of attack between the points A and in the figure.
With increase of the blade element pitch, the straight line AC shifts upward (A’C’). This means that the angle of attack range corresponding to decelerated autorotation increases, and the autorotation margin decreases.
With reduction of the pitch, the straight line AC shifts downward, and the autorotation margin increases.
Since the angles of attack are different for different blade elements, the autorotation conditions for these elements will also be different, and therefore the autorotation margin graph has a somewhat arbitrary nature, i. e., it serves for a qualitative evaluation of this process.
Let us return to the point A on the autorotation margin graph. In our example, it corresponds to ф = 4° and a= 7°30′. At these angles, the autorotation will be steady-state. But how can we obtain an angle of attack a = 7°30′ with an incidence angle ф = 4°?
Since а=ф+Да (see Figure 75b), then Да=а-4. This means that for our example the angle of attack increment caused by the vertical rate of
descent is &x=7°30,-4°=3°30′. Let us find the vertical rate of descent corresponding to this Да if r=0.7; ф=4°; ш=26 rad/sec. It is known that
If the vertical velocity < 7.9 m/sec, then for the given blade
element the angle of attack becomes less than 7°30′, and the autorotation will be decelerated;conversely, if > 7.9 m/sec, the autorotation will be
 accelerated. We must emphasize once again that the words "accelerated autorotation of the element" are arbitrary. They mean that under the given conditions the elemental resultant aerodynamic force vector AR is inclined forward relative to the hub axis and creates a turning moment. The larger the helicopter vertical rate of descent, the larger the angle of attack increment Да, the larger the forward tilt of the force vector AR, and the higher the main rotor rpm will be.
accelerated. We must emphasize once again that the words "accelerated autorotation of the element" are arbitrary. They mean that under the given conditions the elemental resultant aerodynamic force vector AR is inclined forward relative to the hub axis and creates a turning moment. The larger the helicopter vertical rate of descent, the larger the angle of attack increment Да, the larger the forward tilt of the force vector AR, and the higher the main rotor rpm will be.











 If the blade flapping angle does not change azimuthally, the main rotor tip path plane is parallel to the hub rotation plane and the coning axis coincides with the main rotor hub axis. In this case the flapping angle 3 equals the coning angle a^. The coning angle is the angle a^ between the hub rotation plane and the generator of the cone of revolution. It varies from 0° to 10 – 12°.
If the blade flapping angle does not change azimuthally, the main rotor tip path plane is parallel to the hub rotation plane and the coning axis coincides with the main rotor hub axis. In this case the flapping angle 3 equals the coning angle a^. The coning angle is the angle a^ between the hub rotation plane and the generator of the cone of revolution. It varies from 0° to 10 – 12°. If the stick is deflected forward, the axes of the cones-of- rotation of the lifting rotors are tilted forward. The collective pitch of the front rotor is reduced and that of the aft rotor is increased. As the thrust force vectors tilt, there is a change of the thrust force arms relative to the helicopter transverse axis. The result is the creation of a diving moment equal to the difference of the thrust moments of the front and rear rotors.
If the stick is deflected forward, the axes of the cones-of- rotation of the lifting rotors are tilted forward. The collective pitch of the front rotor is reduced and that of the aft rotor is increased. As the thrust force vectors tilt, there is a change of the thrust force arms relative to the helicopter transverse axis. The result is the creation of a diving moment equal to the difference of the thrust moments of the front and rear rotors.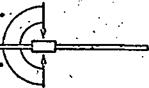

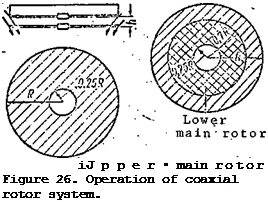 Since its efficiency is less than that of the upper rotor by a factor of two, the additional effective area of the lower rotor is
Since its efficiency is less than that of the upper rotor by a factor of two, the additional effective area of the lower rotor is
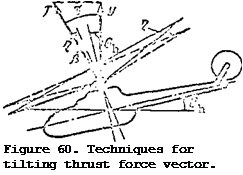 Thus, the thrust force vector forward tilt angle will be equal to
Thus, the thrust force vector forward tilt angle will be equal to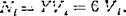
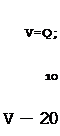 Nt = -2-^- = 245 hP; m/sec: « -22(^,–5- = 147 hp;
Nt = -2-^- = 245 hP; m/sec: « -22(^,–5- = 147 hp; 11:
11: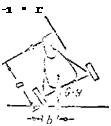 t. r
t. r ‘ t. rt? ,
‘ t. rt? ,