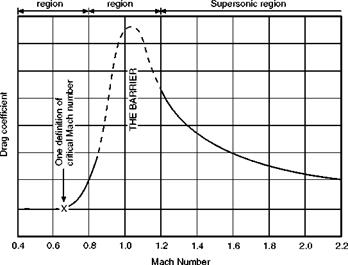
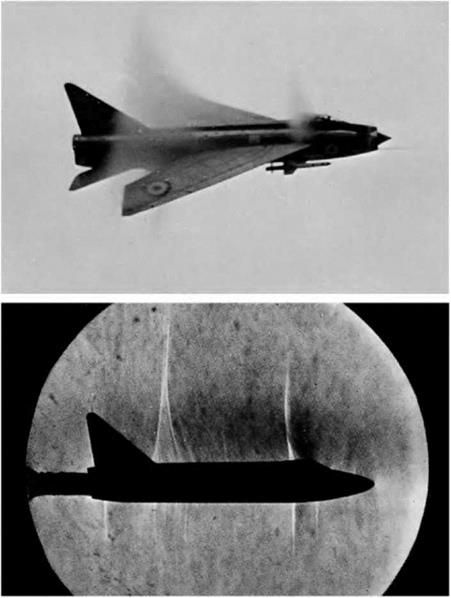
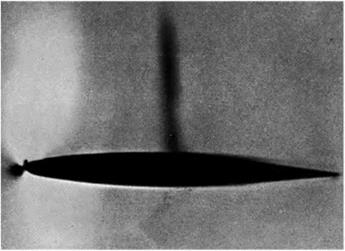
Flight at transonic speeds – drag and power required
There was a time when the prospects of supersonic flight seemed poor owing to the lack of engines with the necessary power to overcome the rapid rise in drag which begins at the critical Mach Number. Even without compressibility effects the drag would rise with the square of the velocity, and the power – which is Drag X Velocity – with the cube of the velocity. The effect of compressibility is to increase these values still further in accordance with Table
11. 2, which shows the approximate figures for a Spitfire.
|
Table 11.2 Power required with compressibility
|
Actually the problem was much more serious than this, if we assumed that the aircraft would be driven by propellers. As was explained in Chapter 4, the efficiency of a good propeller is about 80 per cent at its best, but its ‘best’ is at speeds of 129 to 180 m/s, after which the efficiency falls off very rapidly (although a new generation of propellers has pushed this up) – this happens for various reasons, but chiefly because the propeller tips are the first part of the aircraft to suffer from compressibility. With the high forward speed of the aeroplane combined with the rotary speed of the circumference of the propeller disc, Mach Number troubles begin to occur at aircraft speeds of about 180 m/s, and the result is so disastrous that the power which would have to be supplied to the propeller by the engine in order to attain the speeds given in Table 11.2 would look something more like Table 11.3 a conventional propeller.
Table 11.3 Power required accounting for propellor efficiency
Velocity (m/s) 130 180 230 280 335
Power (kW) to be given to propeller 820 2390 6000 15 000 100 000?
When one looks at these figures one realises why it was that people who knew what they were talking about forecast not so many years ago that it would be a long, long time before we could exceed 300 m/s.
Yet they were wrong. And for one simple reason – the advent of the gas turbine and the first flight of a jet-driven aircraft in 1941. This made all the difference, partly because of the elimination of the propeller and its compressibility problems (it is true that there are similar problems with the turbine blades in the jet engine), but mainly because, the efficiency of the jet increases rapidly over just those speeds, 154 to 257 m/s, when the efficiency of the propeller is falling rapidly. The net result is that whereas the reciprocating- engine-propeller combination requires nearly twenty times as much power to fly at 500 compared with 250 knots, the jet engine only requires about five times the thrust, and it is thrust that matters in a jet engine. Further, the weight of the jet engine is only a small fraction of that of the reciprocating-engine- propeller combination, and at this speed even the fuel consumption is less.
Maybe the prophets ought to have foreseen the jet engine – but they didn’t, at least not within anything like the time during which it actually appeared. Of course, there were good reasons too why they didn’t foresee it, for no metal could then possibly stand up to the temperatures of the gas turbine blades.
The jet engine, then, was the first step in solving the problem of high-speed flight. And while on the subject of engines, the rocket system of propulsion takes us even a step further and no one now, who knows the subject dares predict the limits of speed that may be reached with rockets – outside the atmosphere there really isn’t any limit.
As hinted above, it is interesting to note that lately the wheel looks like turning a full circle. Improvement in propeller design means that the tip problems can be largely overcome and a gas turbine/propeller combination promises better efficiency in the future than a turbojet at transonic speeds.