Case Study: SD7003

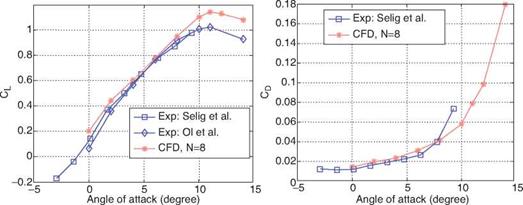
Lian and Shyy [117] studied the Reynolds number effect with a Navier-Stokes equation solver augmented with the eN method. The lift and drag coefficients of the SD7003 airfoil against AoA are plotted in Figure 2.6. This figure vividly shows the good agreement between the numerical results [117] and the experimental measurements by Ol et al. [134] and Selig et al. [88]. Both the simulation and the mea­surement by Ol et al. [134] predict that the maximum lift coefficient happens at the AoA = 11°. Close to the stall AoA, the simulations over-predict the lift coefficients.

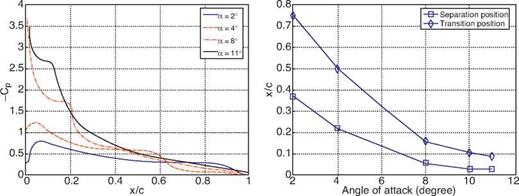
As the AoA increases, as illustrated in Figure 2.7, the adverse pressure gradient downstream of the point of suction peak becomes stronger and the separation point moves toward the leading edge. The strong pressure gradient amplifies the distur­bance in the separation zone and prompts transition. As the turbulence develops, the increased entrainment causes reattachment. At an AoA of 2°, the separation position is at around 37 p of the chord length, and transition occurs at 75 percent of the chord length. A long LSB forms. The plateau of the pressure distribution shown in Figure 2.7a is characteristic of an LSB. As seen in Figure 2.7b the bubble length decreases with an increase in the AoA.

Lian and Shyy [117] compared computed shear stress with experimental mea­surements by Radespiel et al. [135], using the low-turbulence wind tunnel and the

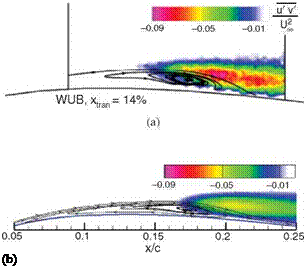
water tunnel because of its low-turbulent nature. Radespiel et al. [135] suggested that large values of critical N factor should be appropriate. As shown in Figure 2.8, the simulation by Lian and Shyy [117] with N = 8 shows good agreement with measurements in terms of the transition position, reattachment position, and vortex core position. It should be noted that, in the experiment, the transition location is defined as the point where the normalized Reynolds shear stress reaches 0.1 percent and demonstrates a clearly visible rise. However, the transition point in the simu­lation is defined as the point where the most unstable TS wave has amplified over a factor of eN. This definitional discrepancy may cause some problems when we compare the transition position. In any event, simulations typically predict notice­ably lower shear-stress magnitude than the experimental measurement. Recently, LES simulations of an SD7003 rectangular wing at the Reynolds number of 6×104 were performed by Galbraith and Visbal [136] and Uranga et al. [137]; they discussed transition mechanisms based on instantaneous and phase-averaged flow features and

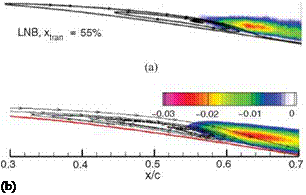
Figure 2.8. Streamlines and turbulent shear stress for the AoA = 4°. (a) Experimental measurement by Rade – spiel et al. [135] and (b) numerical sim­ulation with N = 8 by Lian and Shyy

[117].

turbulence statics. Moreover, using time-resolved particle image velocimetry (PIV), Hain et al. [138] studied the transition mechanism in the flow around an SD7003 wing at Reynolds number of 6.6 x 104; they claimed that the TS waves trigger the ampli­fication of the KH waves, which explains why the size of the separation bubble is affected by the magnitude of the TS waves at separation.

As the AoA increases, both the separation and the transition positions move upstream, and the bubble shrinks. The measurements at the AoAs of 8° and 11° are performed in the water tunnel with a measured free-stream turbulence intensity of 0.8 percent. At the AoA = 8° the simulation by Lian and Shyy [117] predicts that the flow goes though transition at 15 percent of the chord, which is close to the experimental measurement of 14 percent. The bubble covers approximately 8 percent of the airfoil upper surface. The computational and experimental results for the AoA of 8° are shown in Figure 2.9. With the AoA of 11°, the airfoil is close to stall. The separated flow requires a greater pressure recovery in the laminar bubble for reattachment. Lian and Shyy [117] predicted that flow separates at 5 percent of the chord and that the separated flow quickly reattaches after it experiences transition at the 7.5 percent chord position, whereas in the experiment transition actually occurred at 8.3 percent. This quick reattachment generally represents the transition-forcing mechanism. Comparison shows that the computed Reynolds shear stress matches the experimental measurement well (see Fig. 2.10).

For low Reynolds number airfoils, the chord Reynolds number is a key parame­ter used to characterize the overall aerodynamics. Between the separation position and the transition position, as shown in Figure 2.11a, the shape factor H and the momentum-thickness-based Reynolds number increase with the chord Reynolds number. As shown in Figure 2.11b the effective airfoil shape, which is the superim­position of the airfoil and the boundary-layer displacement thickness, has the largest camber at the Reynolds number Re = 4 x 104. This helps explain why the largest lift coefficient is obtained at that Reynolds number (see Fig. 2.11c). The camber decreases significantly when the Reynolds number increases from 4 x 104 to 6 x 104,

Figure 2.9. Streamlines and turbulent shear stress for the AoA = 8°. (a) Exper­imental measurement by Radespiel et al. [135] and (b) numerical simulation with N = 8 by Lian and Shyy [117].

but does not show considerable change when the Reynolds number increases further. Therefore one does not observe much increase in the lift coefficient even though the LSB length is shorter at higher Reynolds numbers. One can conclude from Figure 2.11d that the enhancement of lift-to-drag ratio is mainly due to the reduction of friction drag at high Reynolds numbers. As the Reynolds number increases, the form drag does not vary as much as does the friction drag.